Regular polychoron compounds
"I'm a Platonist - a follower of Plato - who believes that one didn't invent these
sorts of things, that one discovers them. In a sense, all these mathematical facts are
right there waiting to be discovered."
H. S. M. Coxeter.
As in the case of polygons and polyhedra, stellating or faceting a polychoron can result
either in a star polychoron or a regular compound. There are, in total, 36 regular and
16 partially regular polychoron compounds. The fully regular compounds must have
regular "cells" and vertex figures; the difference is that either can be a regular
polyhedron compound as well, in the discussion below we'll call attention to such cases.
Like the regular star polyhedra, they must be stellations and facetings of regular convex
forms because they must also be symmetric around each vertex and around each cell. For the
same reason, the constituent polychora must also be regular.
The partially regular compounds also consist of regular polychora, but they are
stellations or facetings of a regular convex polychoron, but not both. In Fig. 5.4,
we have already seen two examples of the latter class:
- The Compound of two Tesseracts can be represented by the vertex-first model of the
Tesseract. It is cell-regular, i.e., its 16 Cubic cells are in the same 3-D hyperplanes as
those of the 16-cell, making it a stellation of that polychoron. It is not vertex regular:
it has 24 vertices, 8 of each are common to both Tesseracts, eight touch only one of the
Tesseracts, and another eight touch only the second Tesseract; it is therefore not a
faceting of any regular convex polychoron.
- Its dual, the Compound of two 16-cells, can be represented by the cell-first model of
the 16-cell. By the rules of duality, this compound is vertex-regular, i.e. its 16
vertices are those of the Tesseract, making it a faceting of that polychoron. It is not
cell regular: only 8 of its 24 cell planes are common to both 16-cells; it is therefore
not a stellation of a regular convex polychoron.
There are nine additional Zomable
compounds, all of them are fully regular. Below we show all their Zomable projections.
Triality
Before proceeding, we present a brief explanation of the concept of Triality. In the case of duality in 3
dimensions, one can, by the definition of duality, scale and rotate two concentric dual
polyhedra A and B in such a way that each edge from A meets a perpendicular edge of B (see
examples in Figs. 3.3a, b and c). If A and B are regular, their edges meet at their
midpoints, the black balls in Figs. 3.3a, b and c. Those two edges form a plane that is
perpendicular to a third line joining the midpoint to the centres of A and B. Rectifying A
and B results in the same rectified polyhedron (Fig. 3.4).
For the duality of two polychora A and B in 4 dimensions, each edge of A is perpendicular
to a face of B, i.e., the perpendicular elements are not identical. In the case of
Triality in 4 dimensions, the situation is more symmetrical: the edges of three
concentric "trial" polychora meet at right angles. A set of three 16-cells is the only
regular case. Because the three 16-cells are regular, the 3 edges (one from each of the
three 16-cells) meet at their midpoint. These edges are in a 3-D "plane" that is itself
perpendicular to a fourth direction, the line joining that midpoint to the centre of the
three 16-cells. Rectifying all three polychora results in the same rectified polychoron,
the regular case being the 24-cell, whose vertices coincide with the previous edge
midpoints.
The same applies to projections: if we pick three "trial" projections of the 16-cell and
rectify them, we obtain an invariant projection of the 24-cell. In this site, we show a
triad of such trialities. The first we met already in Fig. 5.4: the vertex-first and two
cell-first projections of the 16-cell have the same rectification (the model of the
24-cell in Fig. 5.5a). The other two are shown in Figs. 7.1a (also 5.5b) and b below.
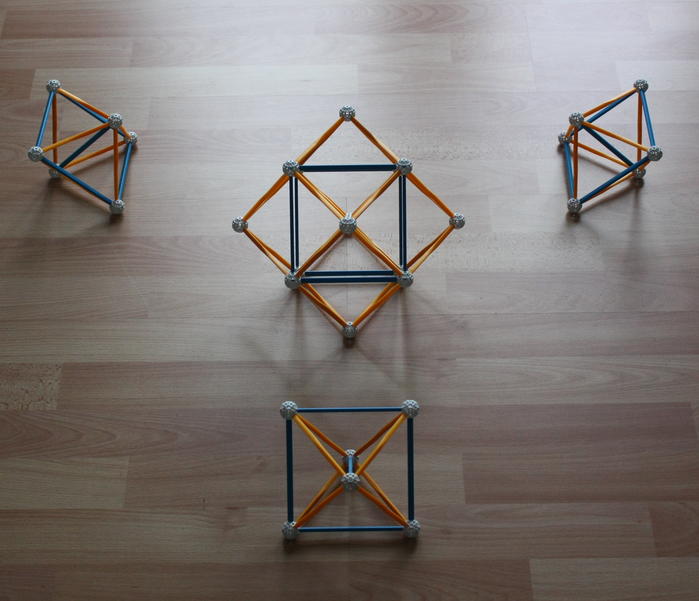
Fig. 7.1a: Three edge-first projections of the 16-cell, and their rectification, the
pyritohedric projection of the 24-cell.
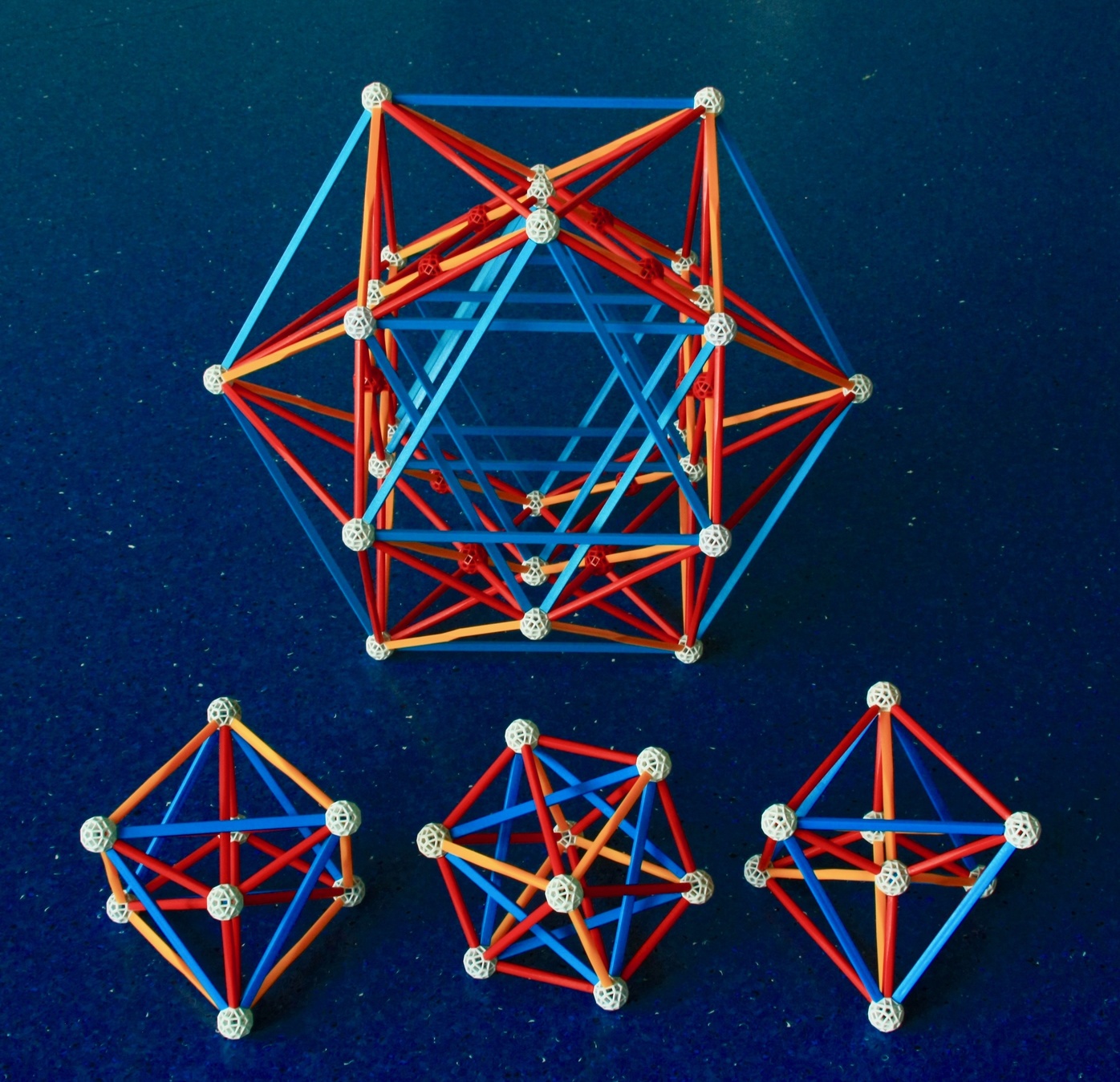
Fig. 7.1b: Bottom: The three "prismatic" projections of the 16-cell (*) and their
rectification, the prismatic projection of the 24-cell.
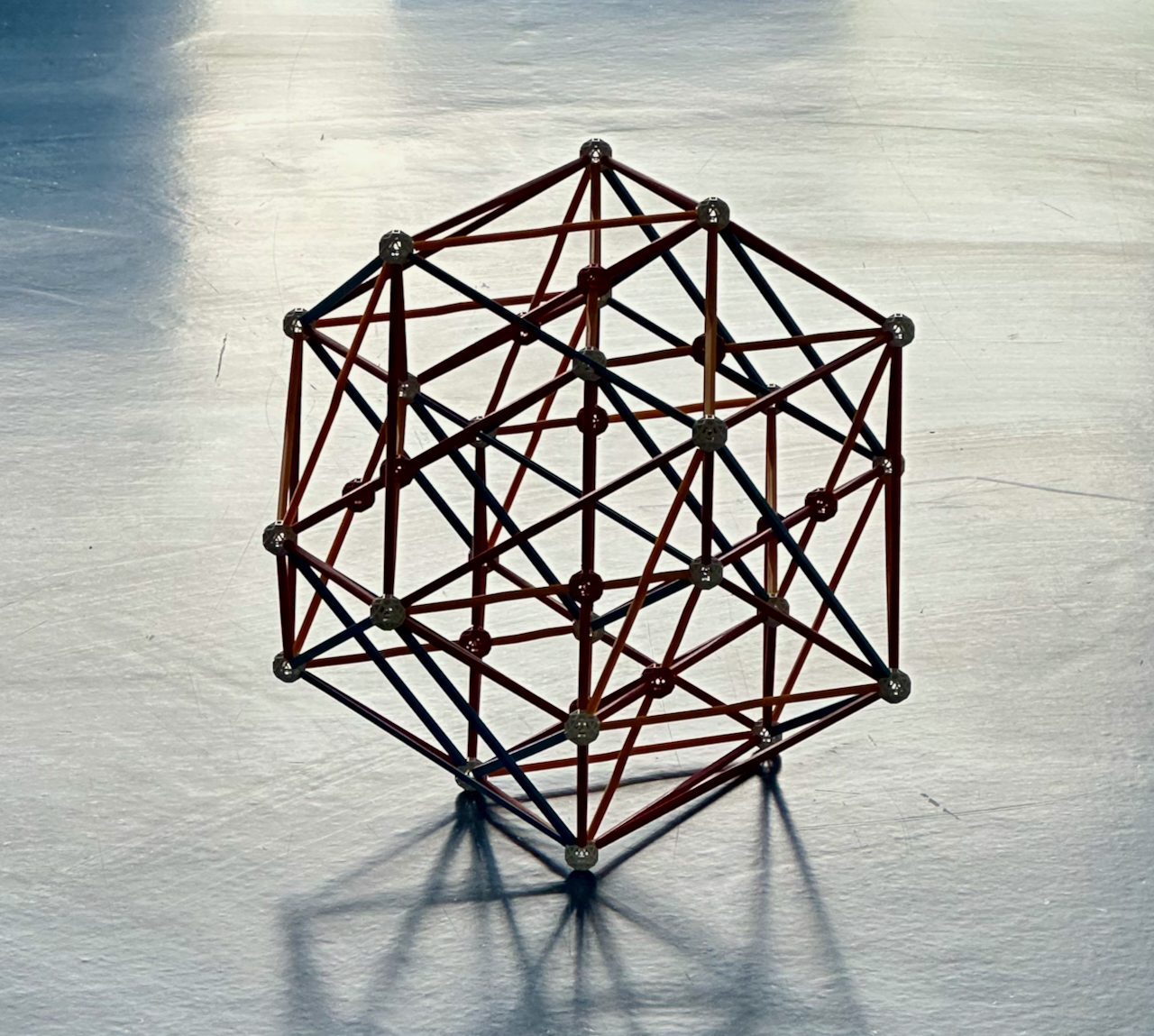
Fig. 7.1c: 3-fold "ghost symmetry" in the prismatic projection of the 24-cell.
Fig. 7.1a shows the edge-first projections of the 16-cell that are (very) loosely aligned
along 3 perpendicular orthogonal axes in 3-d space. The central ``edge'' is actually one
of the equatorial Squares of the 16-cell projected edge-first, so that two edges are
superposed and two others reduced to a point. This Square is perpendicular to the outer
undistorted (blue) Square. Rectifying these three projections, we obtain the vertex-first
projection of the 24-cell in the middle. This is the "pyritohedric" projection we have
seen in Fig. 5.5b.
Fig. 7.1b shows the three prismatic projections of the 16-cell. All are built with the
same set of parts: 8 connectors, 6 R2, 6 R1, 6 Y2, and 6 B2 struts (according to David
Richter, this equality is itself a consequence of the triality). Rectifying all three
projections results in the same projection of the 24-cell (*), also known as its
"prismatic" projection, this is shown by the larger model above. This model shows all 24
real vertices, 96 edges, 96 Triangular faces and 24 Octahedral cells separately. The edges
are, theoretically, represented by 24 R3, 24 R2, 24 Y3 and 24 B3 struts, except for edge
intersections (represented by the 12 red balls), which do not happen in 4 dimensions, only
in this projection. These divide 12 R3 and 12 Y3 struts into pairs of R2 and R1, Y2 and Y1
struts.
The prismatic projection of the 24-cell has axes of apparent (6-fold rotational) symmetry.
This is known as "ghost symmetry", a phenomenon that will be discussed in more detail
below. In Fig. 7.1c, we're looking along one of these axes.
See the section on Coxeter-Dynkin diagrams
for more on triality. For a rigorous treatment, see David Richter's Triality with the
Zometool page.
Facetings of the 24-cell
As mentioned in the derivation of the 24-cell, it has the vertices of three 16-cells. We
can therefore make a regular compound of thee 16-cells with the same vertex arrangement of
the 24-cell, which is therefore its faceting. In the following Figures (7.2a, b and c), we
show on the right three projections of this compound, each of them a superposition of 3
"trial" projections of the 16-cell that appeared, respectively, in Figs. 5.4, 7.1a and b.
The real vertices of the compound are represented by the white balls. The edge midpoints,
where as we mentioned above they intersect by threes at right angles to each other, are by
analogy with Figs. 3.3a, b and c represented by the black balls. These false vertices have
the same arrangement as the real vertices of the rectifications of these projections, the
projections of the 24-cell on the left.
Importantly, there is a stellation operation, greatening, that transforms a 24-cell
into a Compound of three 16-cells. This operation stellates each Octahedral cell of the
24-cell into a "Stella octangula" (Fig. 4.5b) in the same 3-D ``plane", with each
Tetrahedron in the pair belonging to a different 16-cell. Each Triangular face is replaced
by a dual Triangular face in the same 2-D plane, with each edge being replaced by a
parallel one that is twice as long (Fig. 4.5a). Thus, for each strut in a Zomable
projection of the 24-cell on the left there is, within the corresponding greatening on the
right, a parallel set of two struts of the same colour and size in a straight line
connected by a black ball. Therefore, if a projection of the 24-cell is Zomable, then
there is a Zomable projection of the Compound of three 16-cells with the same strut
colours. This will have important consequences below.
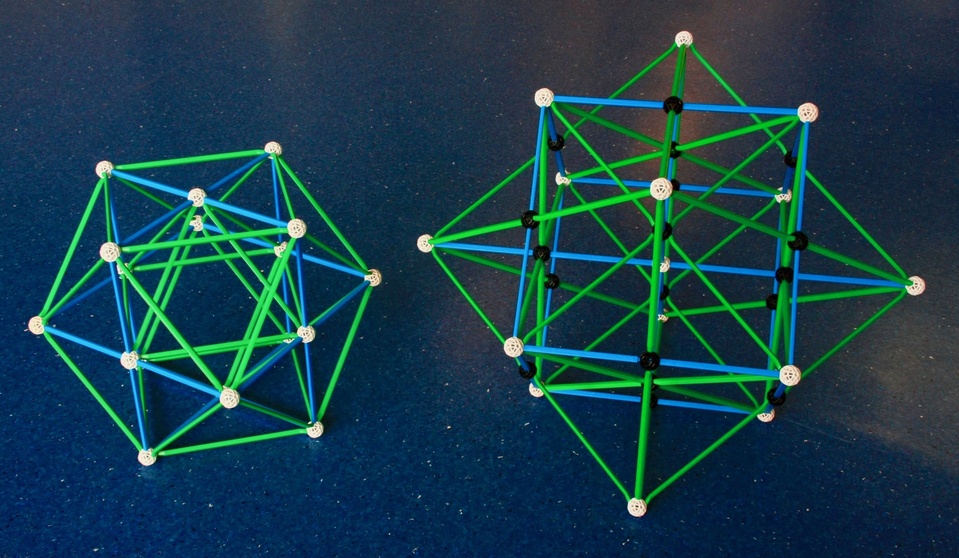
Fig. 7.2a: Left: The Octahedral projection of the 24-cell; right: the Octahedral
projection of the Compound of three 16-cells.
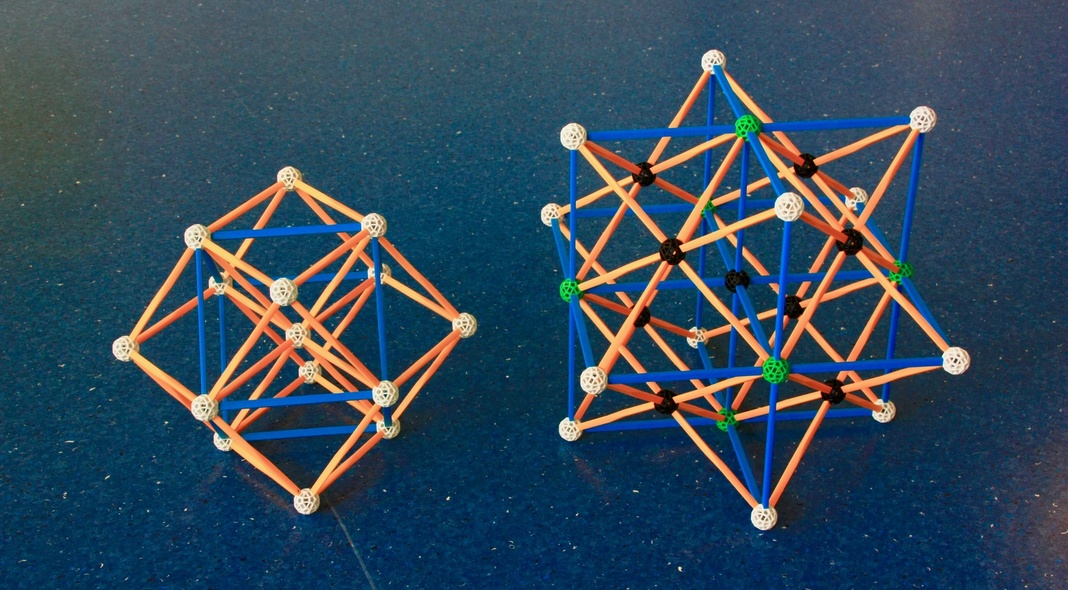
Fig. 7.2b: Left: The pyritohedric projection of the 24-cell; right: the pyritohedric
projection of the Compound of three 16-cells.
Combining one vertex-first projection of the 16-cell in Fig. 5.4 with the projection of
the Compound of two 16-cells in that Figure, we obtain, in Fig. 7.2a, the Octahedral
projection of the Compound of three 16-cells. Since the projection of the 24-cell to its
left has a Cuboctahedral envelope; its stellation's envelope is the first stellation of
the Cuboctahedron: the composite of a Cube and a Octahedron in Fig. 3.3b.
Superposing the 3 orthogonal 16-cells in Fig. 7.1a, we obtain, in Fig. 7.2b, a second
Octahedral projection of the Compound of three 16-cells, its "pyritohedric" projection.
Since the 24-cell to its left has the Rhombic dodecahedron as its outer envelope; its
stellation's envelope is Escher's solid (Fig. 4.10b).
This projection is centred on an intersection of three orthogonal blue lines, an
undistorted orthogonal 3-edge intersection. However, since each blue edge is a central
Square of the edge-centred 16-cells in Fig. 7.1a that has been projected into a line, the
central black node actually represents two 3-edge intersections, one closest to us in 4-D,
the other the farthest. The green balls represent the superposition of two real vertices
(of one of the central Squares) and a midpoint intersection of three edges, from each of
the two outer undistorted Squares and the foreshortened edge of the central Square.
At the centre of the projection of the Compound of three 16-cells in Fig. 7.2a are two of
the vertices of one of the 16-cells; each of these lies "above" two undistorted
Tetrahedral cells from each of the other two 16-cells, the greatening of the ``central''
undistorted Octahedral cells of the 24-cell A on the left. Thus the vertices of this
Compound of three 16-cells lie above the 3-D planes of A, which means that they are the
vertices (and thus the compound is a faceting) of the dual 24-cell, B.
The projections reflect this: the arrangement of true vertices in the projection of the
Compound of three 16-cells in Fig. 7.2a is the same as in the projection of the 24-cell in
Fig. 7.2b (which is the dual of the projection of the 24-cell in Fig. 7.2a); conversely
the arrangement of true vertices in the projection of the Compound of three 16-cells in
Fig. 7.2b is the same as in the projection of the 24-cell in Fig. 7.2a. This distinction
between a 24-cell and its dual is important: The existence of two dual sets of 24-cell
vertices (and the corresponding existence of two dual sets of 24 3-D cell planes) is a
consequence of the aforementioned fact that the Icositetrachoric symmetry has two sets of
12 identical axes of Octahedral symmetry.
Combining the three prismatic projections of the 16-cell in Fig. 7.1b we obtain, in Fig.
7.2c, the prismatic projection of the Compound of three 16-cells (*). The red balls in
both models are edge intersections that appear because of the projection; they are not
real edge intersections in 4 dimensions. This projection shows all true and false vertices
without superposition.
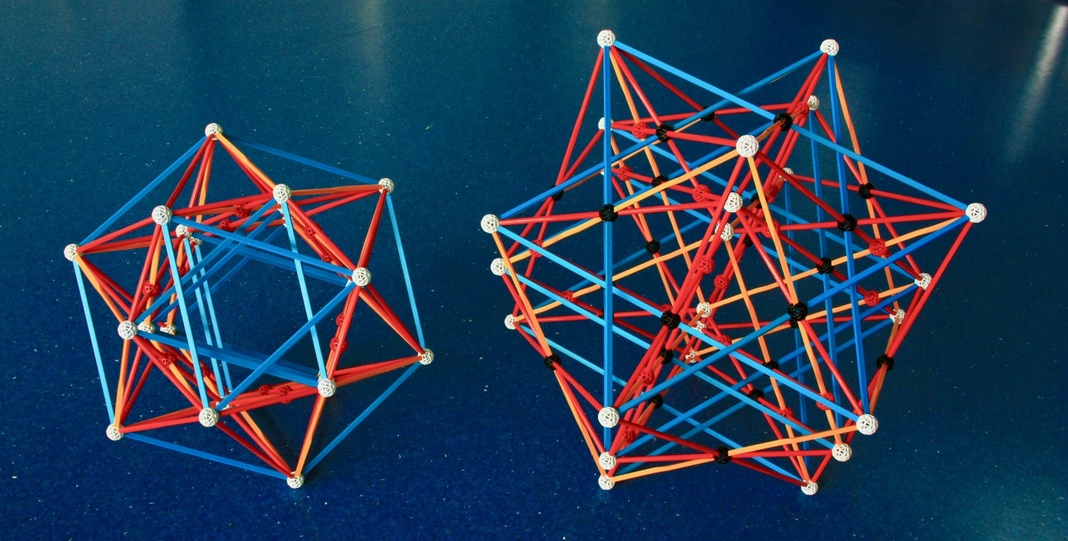
Fig. 7.2c: Left: The prismatic projection of the 24-cell; right: the prismatic projection
of the Compound of three 16-cells.
How to build this projection of the Compound of three 16-cells: Build any of the
prismatic projections of the 16-cell in Fig. 7.1b with either size 2 or size 3 struts as
the largest, with two struts connected by a black ball for each single strut in that
projection in Fig. 7.1b. The models in Fig. 7.2c use size 3 struts. Then build the other
two 16-cells in prismatic projection using the same black balls as edge mid-points. In
this way, you will be able to figure out all the strut intersections. Building the model
on a φ smaller scale requires 6 ultra-short red struts (R00), which are currently sold
by the Zometool company. If you can find size 3 struts, the larger scale is preferable,
otherwise the model looks very dense.
Check also David Richter's
dedicated page, which provides more details on this model.
Since the Compound of three 16-cells is a faceting of the 24-cell, the vertices of its
prismatic projection are also the vertices of the ``anti-prismatic'' projection of the
24-cell, the dual of its prismatic projection in Fig. 7.2c. However, if you try to build
it, you'll find that it is not Zomable (see Fig. 8.2). Its greatening, the anti-prismatic
projection of the Compound of three 16-cells, will have the same vertices as the prismatic
projection of the 24-cell and will have the edge directions of the anti-prismatic
projection of the 24-cell (from the rule mentioned above on greatenings), therefore it is
not Zomable either. The fact that these projections are not Zomable will have important
consequences below.
***
It follows from the properties of the Compound of three 16-cells that its dual, the
Compound of three tesseracts, is necessarily an edge faceting of a 24-cell and a
stellation of its dual 24-cell. The first statement means that it shares all three
Zometool representations of the 24-cell:
- As mentioned in the derivation of the 24-cell, the pyritohedric projection of the
24-cell (Figs. 5.5b, 7.1a and 7.2b) looks exactly like a superposition of the Compound of
two vertex-first Tesseracts and the cell-first projection of the Tesseract in Fig.
5.4.
- Its dual, the Octahedral projection of the 24-cell, looks like three superposed
face-first projections of Tesseracts (see Fig. 7.3).
- Challenge to the reader: Locate the three Tesseracts in the prismatic
projection of the 24-cell (Figs. 7.1b, 7.2c), and build models with the Zometool. How many
models do you need? Are these the dual projections of the 16-cells in Fig. 7.1b or
not?
The identical edge arrangement implies that the 24-cell and Compound of three tesseracts
share the same equatorial polyhedra, a set of 12 Cuboctahedra, which share the former's
Triangular faces and the latter's Square faces.
The second statement, that it stellates the dual 24-cell, can be seen directly from its
pyritohedric projection (Fig. 7.2b), where the Cubic cells appear directly ``under'' the
vertices of the 24-cell, i.e. the Cubic cells are in 3-D planes that are in dual positions
to the cells of the 24-cell being faceted. This will be important for the discussion
below.

Fig. 7.3: Top left: The pyritohedric projection of the Compound of three 16-cells (Fig.
7.2b). Its outer envelope is Escher's solid (Fig. 4.10b).
Bottom left: One of the components of the latter, the edge-first projection of the
16-cell. Its outer envelope is the Yellow dipyramid (Fig. 4.10b).
Bottom right: Its dual, the face-first projection of the Tesseract. That central face is
perpendicular to the central edge of the previous projection of the 16-cell; the 3-d
envelope of this projection is the Yellow cuboid (Fig. 4.4a).
Top right: The dual of the Compound of three 16-cells, the Compound of three Tesseracts.
This projection, the dual of the projection on top right, shares the Zometool
representation of the cell-first projection of the 24-cell in Fig. 7.2a. With some
attention, you can see the 3 face-first Tesseracts in that model. Its 3-D envelope is the
first faceting of the Cuboctahedron (Fig. 4.10c), which is composed of three Yellow
cuboids.
A consequence of these dualities is that the vertex arrangement of the Compound of three
16-cells is exactly the same as for its dual Compound of three tesseracts. The same
happens, therefore, for their cell planes. The same happens therefore to their
projections, as we can see in Fig. 7.3. The same happens therefore to the envelopes of
their projections; Escher's solid (Fig. 4.10b) and its dual, the Compound of three yellow
prisms (Figs. 4.10c), indeed after those two figures we had already noticed their
identical vertex and facial plane arrangements.
The Tesseracts in this compound have a total of 16 × 3 = 48 vertices, twice as much
as the number of vertices of the compound. This implies that each vertex in the compound
is shared by two Tesseracts. Thus, the vertex figure of this compound consists of two
vertex figures of the Tesseract, which are Tetrahedra. Since the Compound of three
tesseracts is an edge-faceting of the 24-cell, the two Tetrahedra of its vertex figure
must be a faceting of the Cubic vertex figure of the 24-cell, i.e., they are arranged as a
Stella octangula (Fig. 4.5b). This has to be, because the dual Compound of three 16-cells
has 24 Stellae octangulae as "cells".
***
No additional regular compounds are edge facetings of the 24-cell: because the 24-cell is
radially equilateral, it can only be edge-faceted by other radially equilateral polychora,
the only one being the Tesseract. By duality, we conclude that there are no additional
regular greatenings of the 24-cell.
We've described the two regular facetings and stellations of the 24-cell in detail because
they are important for understanding what follows. For each compound of n 24-cells below
(with n = 5 and 25), there is a compound of 3n Tesseracts, which is an edge faceting of
the compound of n 24-cells (this will have the Stella octangula as its vertex figure), and
a Compound of 3n 16-cells, which is a greatening (with twice the edge length, but the same
edge directions) of the compound of n 24-cells; this will have n × 24 "Stellae
octangulae" as composite "cells".
The Zomable projections of these compounds shown below are made of multiples of the
pyritohedric and prismatic projections of the 24-cell/Compound of three tesseracts or the
Compound of three 16-cells above, with the prismatic projections being 4 times more
numerous than the pyritohedric projections: the single axis of 3-fold symmetry of a
Prismatic projection coincides with each of the 4 axes of 3-fold symmetry of the
pyritohedric projections. Because the duals of the prismatic projections are not Zomable,
then none of the duals of these projections are Zomable either (see, for instance, Figs.
8.3 and 8.4), even if they represent a Zomable compound, or even of they represent exactly
the same compound (as in the case of the self-dual Compound of five 24-cells).
All of these models suffer from the multiple blue intersections that we found with the
models of the regular star polychora, the reason is that, as we will see later, they share
their edge directions. Like the latter models, all these models are built on a scale φ
times smaller than the corresponding models in David Richter's projects with the
Zometool page, and for the same reasons.
Facetings of the 600-cell
In the case of the Icosahedron, there are only three facetings: three of the regular star
polyhedra. We have already seen above, the 600-cell also has several regular facetings:
nine of the regular star polychora.
However, and unlike the Icosahedron, it also has several facetings that are regular
compounds, all of which share its full Hexacosichoric symmetry.
We start with the Compound of five 24-cells. If you followed attentively the construction
of the 600-cell from the 24-cell, you can check that the last vertices to be added (the 24
pyramidal apices) have the same arrangement as the vertices of a 24-cell. Again, the
symmetry of the 600-cell implies that there is nothing special about these apices: the 96
vertices of the Snub 24-cell can be decomposed into 4 groups of 24 vertices, each also
with the same arrangement as the vertices of a 24-cell. This means that we can build a set
of five 24-cells with the same vertex arrangement of the 600-cell. A projection, with the
same vertex arrangement as the Icosahedral projection of the 600-cell in Figs. 5.10 and
6.1 is shown in Figs. 7.4a and b.
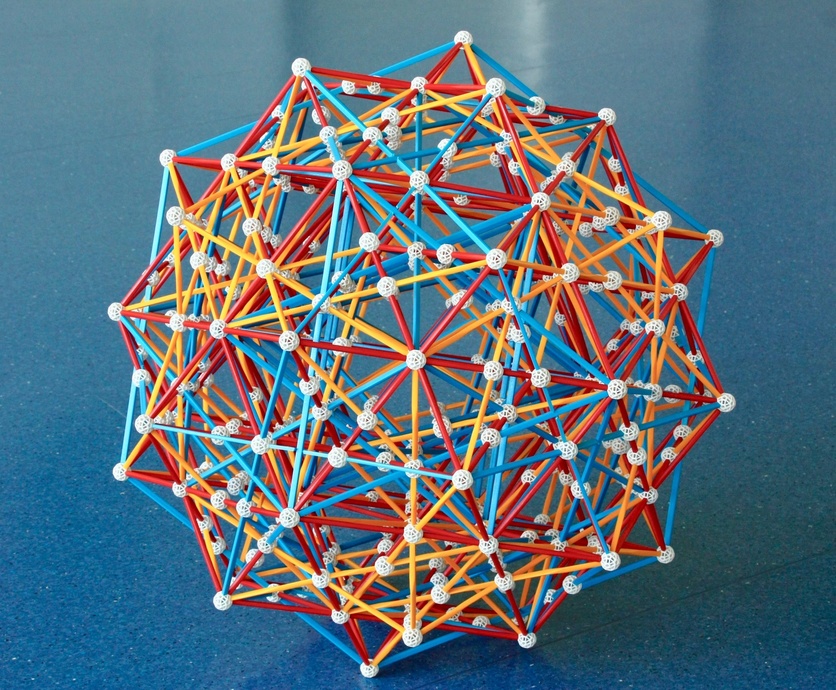
Fig. 7.4a: This model represents a projection of the Compound of five 24-cells, here seen
through one of the 8 directions along the 4 genuine three-fold rotational symmetry axes.
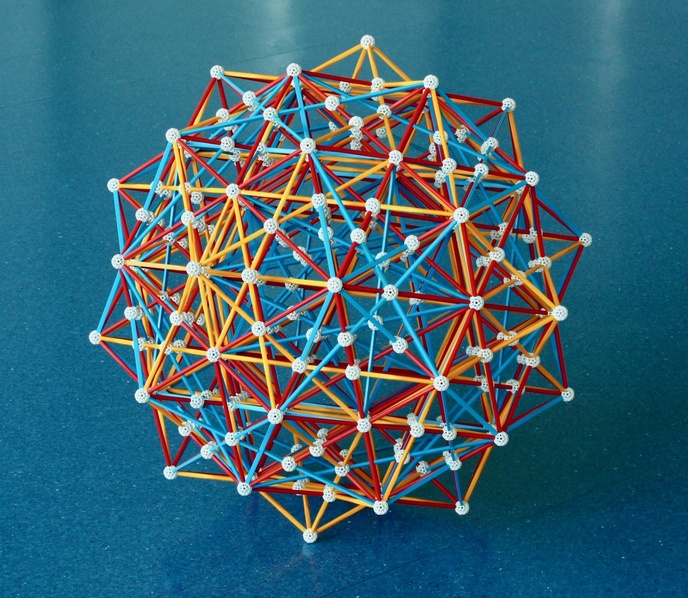
Fig. 7.4b: The same projection as in the previous Figure, now seen through one of 12
special directions, along the 6 Pentagonal "Ghost symmetry" axes (see text). There are an
additional 6 Triangular ghost symmetry axes.
See detailed explanation of this model in David Richter's
dedicated page, which includes a part count.

Fig. 7.4c: The core of the Compound of five 24-cells.
How to build: The model in Fig. 7.4c is the core of the model in Figs. 7.4a and b.
It is nearly identical with a Stellated dodecahedron, with three pais of opposite edges
(in orthogonal directions) removed, the inner eight yellow struts connect the centre to
the inner false vertices where three blue edges cross.
By examining the centre of the prismatic projection of the 24-cell (Figs. 7.1b and 7.2c),
you can see that the struts nearer to the centre are blue, making two Triangles in dual
positions. The four pairs of opposite blue triangles in this model (perpendicular to the
yellow struts) are the starting point for building 4 replicas of the prismatic projection
of the 24-cell; for this having a model of the latter is very handy.
The central yellow struts are the starting point for building a 24-cell in pyritohedric
projection, this must be built at the same scale as that of the prismatic projections (the
blue edges must have the same lengths).
Building the model at this scale has an interesting implication: If we can find some size
3 struts (12 R3 and 12 Y3), we can use each of them to replace a combination of two struts
and one ball where the ball is not strictly necessary (i.e., a ball where there is no
intersection with other edges). Doing this, we can save 48 regular-sized struts and 24
balls compared to the part count in David Richter's page on this model and improve its
appearance.
This regular polychoron compound is one of the very few that are self-dual. Since it is a
faceting of the 600-cell, its dual - in this case itself - is necessarily a stellation on
the 120-cell.
Despite the Icosahedral vertex arrangement, this projection has the pyritohedral symmetry
of the model of the irregular icosahedron and the Snub 24-cell in Figs. 5.7 and 5.8. This
is unusual since the Compound of five 24-cells has full Hexacosichoric symmetry: for most
other similarly symmetric objects, the only Zomable projections have Icosahedral
symmetry. The reason for this is explained in the next
page.
In this projection there are no axes of 5-fold symmetry, as we would naturally find in a
model with Icosahedral symmetry. However, if we project this model into 2 dimensions along
a set of 12 special directions - as in Figure 7.4b - we see again a semblance of 5-fold
symmetry. This is a remnant of the fact that the original compound can be inscribed in the
600-cell. The original paper describing this (Richter and
Vorthmann, 2006) calls this phenomenon "Tenacious Symmetry". However, the name "Ghost
symmetry" has gained wider acceptance, since it is more evocative. It is a widespread
phenomenon, caused by the fact that the 2-D symmetries are rather "small": one can choose
the vertices of a polychoron to project symmetrically into two dimensions, and have an
intermediate 3-D projection that is still highly asymmetric. An extreme example are these two projections
of the 600-cell.
***
This model also represents the Compound of fifteen tesseracts, which is obtained by
edge-faceting the 24-cells of the previous compound. As discussed above, for the Compound
of three tesseracts that results from each of those 5 24-cells, the 3-D planes of the
Cubic cells are in the 3-D planes of the dual 24-cell. Therefore, the 15 × 8 = 120
Cubic cells of the compound of fifteen Tesseracts are in the same planes as the the cells
of the dual of the Compound of five 24-cells, which is of course another Compound of five
24-cells. As we saw above, the latter is a stellation of the 120-cell, so the same applies
to the Compound of fifteen tesseracts.
***
Since the Compound of five 24-cells is self-dual, its edge faceting (the Compound of
fifteen tesseracts in Figs. 7.4a and b) is the dual of its own greatening, the Compound of
fifteen 16-cells. A projection of the latter is shown in Figs. 7.5a and b. Like its dual,
it is a faceting of the 600-cell and a stellation of the 120-cell.

Fig. 7.5a: A projection of the Compound of fifteen 16-cells. Here we see the model through
one of the 4 three-fold rotational symmetry axes.
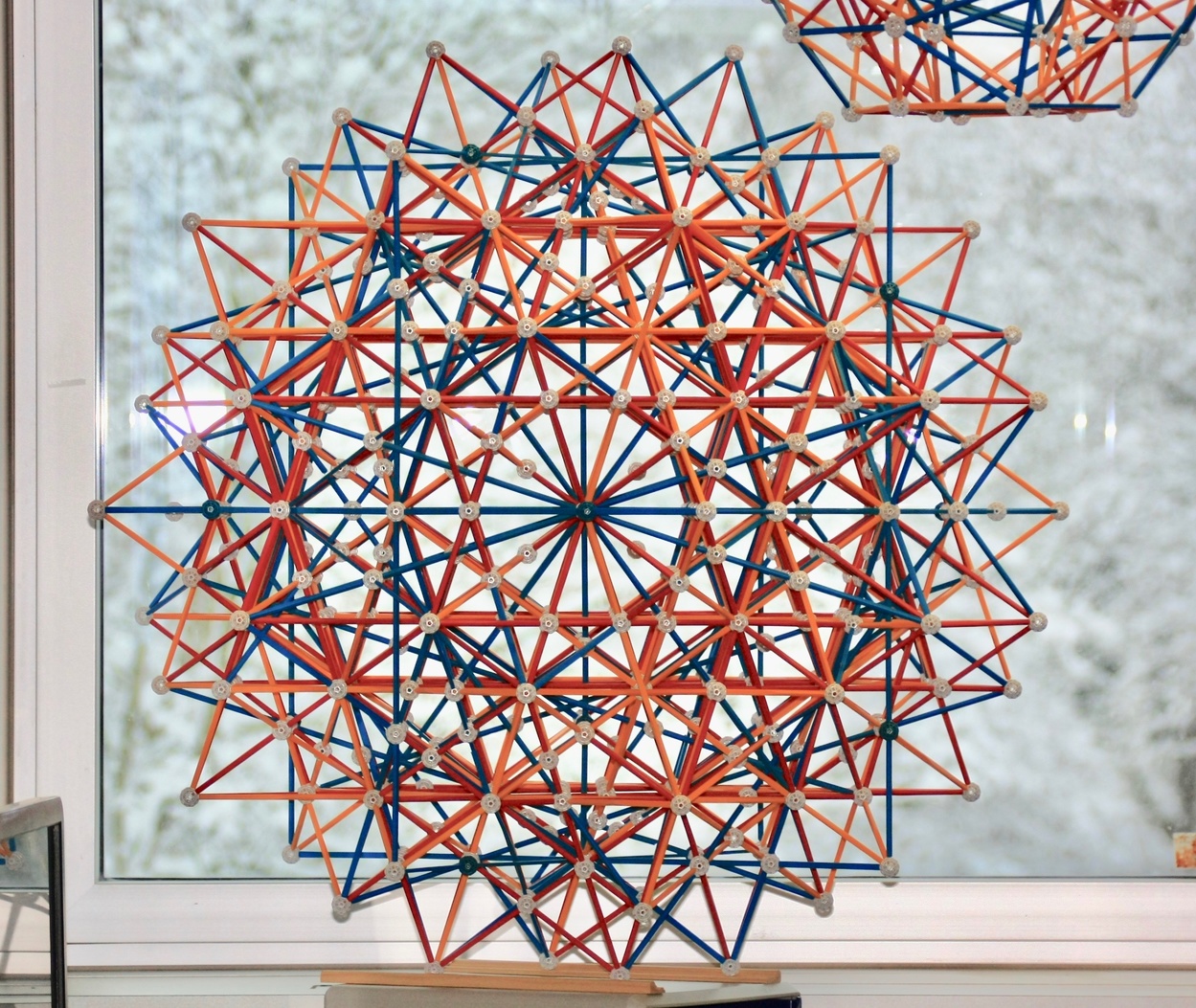
Fig. 7.5b: The same model as in the previous Figure, here seen through one of the 6
Pentagonal "ghost symmetry" axes.
See also David
Richter's dedicated page, which has a detailed description and part count.

Fig. 7.5c: The core of the Compound of fifteen 16-cells.
How to build: By examining the centre of the prismatic projection of the Compound
of three 16-cells (Fig. 7.2c), you can see that the struts nearer to the centre are
yellow, in particular two sets of three radiating from two opposite apexes. The model in
Fig. 7.5c is the core of the model in Figs. 7.5a and b. The outer 4 pairs of opposite
apexes where three yellow struts converge are the starting points for building 4 replicas
of the prismatic projection of the Compound of three 16-cells; for this having a model of
the latter is very handy. Note that at the same time, one has to start building, from the
centre of this model, a projection of the Compound of three 16-cells in pyritohedric
projection (Fig. 7.2b) using a central ball and blue struts (again, having that projection
at hand is useful). Those blue struts must be oriented in a way that is consistent with
the remainder of the model, and the pyritohedric projection must be made at the same scale
as the prismatic projections. Building these projections slowly inside out you will be
able to figure out all the strut intersections. Building this model is a bit tricky
because the Compound of three 16-cells in pyritohedric projection remains disconnected
from the remainder of the model for quite a while!
This model cannot be built on a smaller scale, because it uses the smallest available
yellow struts (Y0). At this scale, we can use size 3 struts (in this case, 72 B3, 48 R3
and 120 Y3) to save 480 regular-sized struts and 240 balls from the part count in David
Richter's page on this model and improve its appearance.
This particular projection is the greatening of the projection of the Compound of five
24-cells in Figs. 7.4a and b, therefore it has the same pyritohedric symmetry, despite the
full Hexacosichoric symmetry of the Compound of fifteen 16-cells. The 24-cell in
pyritohedric projection is greatened into a Compound of three 16-cells in pyritohedric
projection (see Fig. 7.2b), and the four 24-cells in prismatic projection are greatened
into four Compounds of three 16-cells in prismatic projection (see Fig. 7.2c).
However, and uniquely in this projection, its vertex arrangement is not that of the
Icosahedral projection of the 600-cell in Fig. 5.10, but instead the vertex arrangement of
its Tetrahedral projection, shown in Fig. 8.1. This is a 1/5 subset of the vertex
arrangement of the Icosahedral projection of the 120-cell in Fig. 5.11. This reflects a
fundamental fact, that the 120-cell can be faceted by 600-cells, either five or ten
(see Fig. 8.5). We will see a few important consequences of this below.
***
There are four regular compounds of twenty-five 24-cells. Among them, there is a dual pair
with Hexacosichoric symmetry, where one of the compounds is a faceting of the 600-cell
(see Fig. 7.6) and the other is a faceting of the 120-cell (Fig. 8.3). This implies that the
former is also a stellation of the 600-cell and the latter is also a stellation of the
120-cell.

Fig. 7.6: This model of the Icosahedral projection of the Stellated 120-cell and Great
grand 120-cell, also represents the Icosahedral projections of two regular polychoron
compounds: one of the Compounds of twenty-five 24-cells and one of the Compounds of
seventy-five Tesseracts.
Since the twenty-five 24-cells of the compound in Fig. 7.6 have a total of 600 vertices,
they have to coincide by fives with the 120 vertices of the 600-cell. Since the vertex
figure of the 24-cell is a Cube, the vertex figure of this compound is the regular
Compound of five cubes, which facets the Dodecahedral vertex figure of the Stellated
120-cell. Thus the edge arrangement, which is defined by the overall vertex arrangement
(of the 600-cell) and the vertices of the vertex figure (identical to those of the
Stellated 120-cell) is that of the Stellated 120-cell. This means that the Icosahedral
projections of this compound and the Stellated 120-cell are represented by the same edge
model! The 24-cells "fit" because, like the Stellated 120-cell, they are radially
equilateral! The cells of these 24-cells are the 600 Octahedral sections of the Stellated
120-cell.
Furthermore, since the Compound of five cubes shares the edge arrangement of the
ditrigonal polyhedra, which is the vertex figure of the ditrigonal polychora, the Compound
of twenty-five 24-cells shares the latter's face arrangement. Because the
faces in this arrangement are Triangles, the edges of this compound will necessarily trace
its vertex figure, the Compound of five cubes; this is one of the aforementioned edge
sections of the Stellated 120-cell.
The twenty-five 24-cells in the compound have a total of 25 × 96 = 2400 edges, which
is twice the number of edges of the stellated 120-cell. This means that the edges of the
24-cells in this compound coincide in pairs. This is to be expected from the vertex figure
in the Compound of five cubes, each vertex is touched by two different Cubes.
The projection in this Figure can be built from the model of the Compound of five
24-cells in Figs. 7.4a and b by quintuplication (see Fig. 4.1b): To that projection, we
add four more identical ones, each rotated around one of the "ghost symmetry" axes of the
first compound by an additional 72 degrees. The resulting model has Icosahedral symmetry.
***
This model also represents one of the regular Compounds of seventy-five Tesseracts, which
is obtained by edge-faceting the 24-cells of the previous compound. Its dual is therefore
the greatening of the dual Compound of twenty five 24-cells in Fig. 8.3, the Compound of
seventy-five 16-cells in Fig. 8.4. Since this Compound of seventy-five tesseracts is a
faceting of the 600-cell, their 16 × 75 = 1200 vertices must fall in sets of 10 on
the 120 vertices of this compound. Since the vertex figure of the Tesseract is a
Tetrahedron, the vertex figure of this compound is the Compound of ten tetrahedra*,
which is also a faceting of the Dodecahedral vertex figure of the Stellated 120-cell.
As in the previous compound, the 75 × 32 = 2400 edges of the Tesseracts coincide in
pairs. This is to be expected from the vertex figure: in the Compound of ten
tetrahedra, each vertex is touched by two different Tetrahedra.
As discussed above, when we facet a 24-cell to obtain a Compound of 3 Tesseracts, the
latter's cells are in the 3-D "planes" of the dual 24-cell. Thus, the 3-D cell "planes" of
the Compound of seventy-five tesseracts in Fig. 7.6 are identical to those of the dual
Compound of twenty-five 24-cells in Fig. 8.3, which as mentioned above, is a stellation of
the 120-cell. Thus, its 75 × 8 = 600 Cubic cells appear in sets of five in the cell
planes of the 120-cell, i.e., this compound can be seen as a polychoron with 120 Compounds
of five cubes as composite cells, which edges coincident with the edge sections of the
Stellated 120-cell and Compound of twenty-five 24-cells.
The equatorial polyhedra of the Compound of twenty-five 24-cells and the Compound of
seventy-five tesseracts are a set of 25 × 12 = 300 Cuboctahedra, which are
perpendicular to the 300 axes of Tetrahedral symmetry of Hexacosichoric symmetry. Their
equatorial Hexagons coincide with the equatorial Hexagons of the 60 equatorial
Dodecadodecahedra of the Stellated 120-cell/Great grand 120-cell. Since the projection is
made along an axis of Icosahedral symmetry, all equatorial Cuboctahedra are distorted by
the projection, but they are all clearly visible in it.
No additional regular compounds are edge facetings of the Stellated 120-cell: because the
latter is radially equilateral, any such compounds can only consist of radially
equilateral polychora - the 24-cell and Tesseract. Thus, the list of regular edge
facetings of the Stellated 120-cell is complete.
* When discussing the Compound of three tesseracts above, we said that other
compounds of 3 n Tesseracts also have the Stella octangula as vertex figures. This is
still true in this case, since the Compound of ten tetrahedra can be thought of as a
Compound of five Stellae octangulae.
***
In the facetings of the 600-cell above, we used edge arrangements that result from
connecting each vertex of the 600-cell to vertex section 1 in Table 3 (Fig. 6.1), to
vertex section 2 in Table 3 (Figs. 6.2 a and b, Fig. 7.6) and section 5 in Table 3 (Figs.
6.3a and b). What happens if we try to connect the vertices with vertex sections 3, 4, 6
or 7 in Table 3? Do we get any additional facetings of the 600-cell?
The answer is exactly one: the dual of the Compound of seventy-five tesseracts in Fig.
7.6, the Compound of seventy-five 16-cells in Fig. 8.4. The edges of this compound connect
each vertex to vertex section 4 in Table 3. This compound is shown in the next page because, as we'll see there,
this is the only faceting of the 600-cell that is not Zomable.
The reason for this single additional object is the following: Given the central symmetry
of their vertex figures, any regular facetings of the 600-cell (polychora or compounds)
must have rectifications (or compounds of rectifications) of regular polyhedra as their
equatorial polyhedra, furthermore, these must facet the Icosidodecahedron, which is the
equatorial polyhedron of the 600-cell. All such objects are depicted in Fig. 4.12: no
others are possible because the equatorial polygons in Fig. 4.12 already are perpendicular
to the three types of axes of Icosahedral symmetry. The only such faceting in that model
that has not been used as an equatorial polyhedron in previous models is the Compound of
five octahedra; this is the equatorial ``polyhedron" of the Compound of seventy-five
16-cells in Fig. 8.4. Since there are no additional such facetings, the list of facetings
of the 600-cell is complete.
The equatorial polyhedra of the compound of seventy-five 16-cells in Fig. 8.4. are a set
of 75 × 4 = 300 Octahedra. They are perpendicular to the 300 axes of Tetrahedral
symmetry of the Hexacosichoric symmetry.
Facetings of the 120-cell
As we've seen, the Dodecahedron has only one regular star polyhedron (the Great stellated
dodecahedron) as a faceting, but three regular compounds: the Compounds of five and ten
Tetrahedra, and the Compound of five Cubes. The situation is analogous for the 120-cell:
only one regular faceting (the Great grand stellated 120-cell) but many regular compounds,
which have its vertex sections (listed in Table V of Coxeter 1973) as cells. Its regular
facetings can be divided in three main classes:
- The Great grand stellated 120-cell, the only regular polychoron in this
list,
- The largest group (39 regular and partially regular compounds) results from the fact,
mentioned after Fig. 7.5b, that we can facet a 120-cell with 5 or 10 600-cells (see Fig.
8.5). This has a major implication: all facetings of the 600-cell (either 9 of the 10
regular star polychora or the regular polychoron compounds just mentioned above) can then
be used to form new regular compounds that are also facetings of the 120-cell. Some of
these compounds, like the Compounds of five and ten 600-cells themselves, are
partially regular facetings of the 120-cell; they and their duals (which are
partially regular stellations of the 600-cell) are also included in this group,
- The last group (3 regular compounds) arises from the fact that, unlike the 600-cell,
the 120-cell can be faceted by 5-cells. This is analogous to the fact that the
Dodecahedron can be faceted with Tetrahedra.
This list implies that the vertex arrangement of the 120-cell includes the vertex
arrangements of all other regular polychora as subsets! This is very different from the
Dodecahedron, which cannot be faceted by the Icosahedron and its facetings, or Octahedra
for that matter. This implies, by duality, that the 3-D cell planes of all regular
polychora are subsets of the 3-D cell planes of the 600-cell.
In Figs. 6.4a and b, we showed a model of the single member of the first class. In Figs.
7.7a, b, c, d and 7.9a, b, we present the single regular compounds from the second and
third classes that are Zomable (for an explanation on why this is the case, look here). It's nice that there are Zomable members of the
three classes! However, as we see in those Figures, these models are extremely complex and
require many parts; this is an unavoidable consequence of the large number of vertices of
the 120-cell (600). The outer vertices have the same separations as the model in Figs.
6.4a and b.
The compounds represented by the Zometool models below have full Hexacosichoric symmetry;
however, this is not true for all facetings of the 120-cell (like the Compound of five
600-cells, see Fig. 8.5).
***
We start with the single Zomable faceting of the second class, the regular Compound of
seventy-five 16-cells with full Hexacosichoric symmetry.

Fig. 7.7a: The Icosahedral projection of the Compound of seventy-five 16-cells that facets
the 120-cell and has full Hexacosichoric symmetry.

Fig. 7.7b: The model is seen here from a 3-fold symmetry axis, and without
counter-illumination.

Fig. 7.7c: When the Sun is at a good elevation, these models cast really nice shadows!
Note the small bit of light right at the centre of the shadow: sunlight shone through all
the pentagonal holes of the nine balls in one of the six central axes of 5-fold symmetry
of the model. This is proof that the parts in the model are aligned with very good
precision.

Fig. 7.7d: Same as Fig. 7.7c, but at at different time of the day, with the Sun shining
through a 3-fold symmetry axis.
Unlike the picture of the models, which are perspective projections, these shadows are
almost orthographic projections of the 3-D model to 2-D, but also from the 4-D object to
2-D. They are not fully orthographic because the vectors used in the projection - the
Sunbeams - are not perpendicular to the 2-D surface (the floor of my living room);
however, like orthographic projections they are affine transformations. Apart from that,
they are quite beautiful!
See also David Richter's
page on this compound.

Fig. 7.7e: The core of the Compound of seventy-five 16-cells.
How to build: By examining the centre of the prismatic projection of the Compound
of three 16-cells (Fig. 7.2c), you can see that the struts nearer to the centre are
yellow, in particular two sets of three radiating from two opposite apexes. The model in
Fig. 7.7e is the core of the model in Figs. 7.7a, b, c and d. The 20 pairs of outer apexes
where three yellow struts converge are the starting points for building 20 replicas of the
prismatic projection of the Compound of three 16-cells; for this having a model of the
latter is very handy. The 30 blue struts radiating from the centre (from all the
rectangular holes of the central node) are the starting points for building five Compounds
of three 16-cells in pyritohedric projection (Fig. 7.2b), these must be built at the same
scale as the prismatic projections. Building these projections slowly inside out you will
be able to figure out all the strut intersections.
The model cannot be built on smaller scale because it uses many B0 and Y0 struts. At this
scale no size 3 struts are necessary!
This compound is Zomable because it is the greatening of the Compound of twenty-five
24-cells in Fig. 7.6. Since all stellations preserve the 3-D cell "planes", then this
compound is also a stellation of the 600-cell. Its dual is the edge faceting of the dual
Compound of twenty-five 24-cells in Fig. 8.3, another Compound of seventy-five
Tesseracts.
Regarding this particular projection, it can be derived from the projection of the
Compound of fifteen 16-cells in Figs. 7.5a and b by quintuplication. Each of those five
projections facets a Tetrahedral projection of the 600-cell, five of which cover all the
vertices of the Icosahedral projection of the 120-cell in Fig. 5.11.
The equatorial polyhedra of this compound of seventy-five 16-cells are a set of 75 ×
4 = 300 Octahedra. They are perpendicular to the 300 axes of Tetrahedral symmetry of the
Hexacosichoric symmetry of the compound. Since the projection is made along an Icosahedral
symmetry axis, all of these equatorial Octahedra are distorted. However, for each of the
fifteen 16-cells in pyritohedric projection (see Fig. 7.1a), we can see an undistorted
equatorial Square. This is perpendicular to another Square of the same 16-cell, which is
projected as a blue line segment (see Fig. 7.1a) and goes through the centre of the
projection. The fifteen blue line segments going through the centre of the projection are
aligned with its 15 axes of 2-fold symmetry, these can be seen well in the model in Fig.
7.7e.
***
Until now, all fully regular compounds we have built were Compounds of n 24-cells (with n
= 1, 5, and 25) and compounds derived from them by edge-faceting (with 3n Tesseracts,
which are represented by the same models) or greatening (with 3n 16-cells). That basically
sums it up. However, the last model below represents something truly different: it is a
compound of 5-cells.
In Fig. 5.3, we saw the vertex/cell first projection of the 5-cell. In the Figure below, I
show three more Zomable projections.
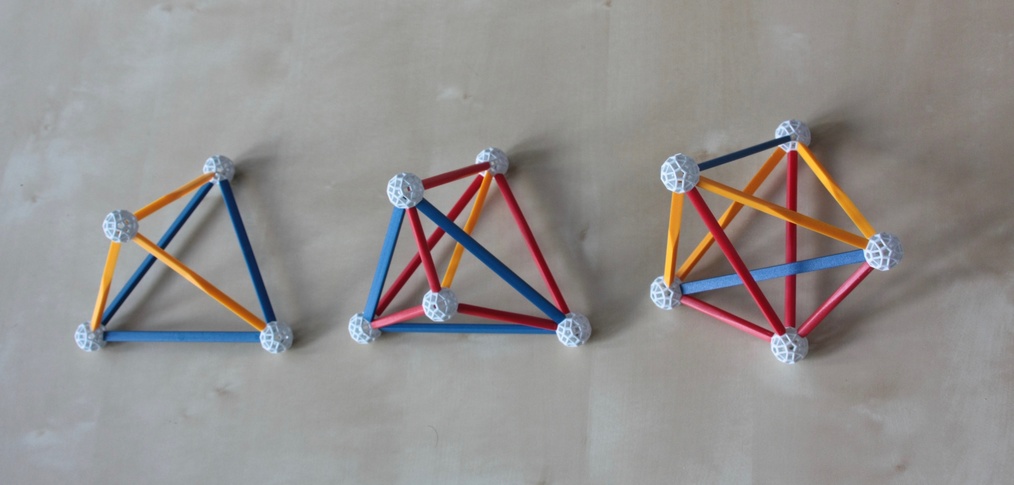
Fig. 7.8: Three Zomable projections of the 5-cell.
In this page I name these from left to right, the "triangular", "prismatic" and
"ghost-symmetric" projections. The latter was found by Scott Vorthmann, the other two were
found later by David Richter. The name for the projection on the right comes from the fact
that it has pentagonal ghost symmetry: from this perspective, we can see a Pentagram
inscribed in a Pentagon. For a more complete description of this projection and its
implications, see Scott's
vZome page on this projection.
With these three projections, we can build a model of the Icosahedral projection of the
Compound of 120 5-cells, this is shown below.

Fig. 7.9a: The Icosahedral projection of the regular Compound of 120 5-cells with
Hexacosichoric symmetry, here seen from a 5-fold symmetry axis.

Fig. 7.9b: Same model as in the previous Figure, seen here from a 3-fold symmetry axis.
The model cannot be built at a smaller scale because it already uses Y0 struts. At this
scale, we can use 180 B3, 120 R3 and 260 Y3 struts; this saves 560 balls and 1120 regular
sized struts and greatly improves the appearance of the model.
This regular polychoron compound is special for several reasons. It is self-dual, a
property it shares only with the Compound of five 24-cells (Figs. 7.4a and b) and the
other two regular 5-cell compounds. Among the latter, it is the only Zomable compound.
Since it is a faceting of the 120-cell, and it is made of 5-cells, it has 600 vertices
with Tetrahedral vertex figures, like the Great grand stellated 120-cell and the
120-cell. This and the fact that it is self-dual implies that it is a stellation of the
600-cell: like the Grand 600-cell, it has 600 Tetrahedral cells located in the same 3-D
"planes" of the 600 Tetrahedral cells of the 600-cell. All of this makes it a 4-D analogue
of the self-dual Compound of five tetrahedra.
The story behind this model is interesting. It is not in David Richter's list of projects with the
Zometool, so I did not know whether the Compound of 120 5-cells was Zomable. However,
when I saw the "ghost symmetric" projection of the 5-cell in Scott
Vorthmann's vZome page on the 5-cell, I wondered whether it can be inscribed in the
Icosahedral projection of the 120-cell (Fig. 5.11). I asked Scott about this, and he found
out that the answer is "yes". Not only that, but the same is true for the other two
projections of the 5-cell in Fig. 7.8.
Even more interestingly, he found that with only those three projections (20 triangular,
40 prismatic and 60 ghost-symmetric) we can cover all the vertices of the Icosahedral
projection of the 120-cell. Thus, there is a Zomable projection of the Compound of 120
5-cells, with the same vertex arrangement as the model in Fig. 5.11 and therefore with the
same Icosahedral symmetry! To demonstrate this, Scott made a very nice vZome model of the
Icosahedral projection of this Compound, and Nan Ma (see star polytope page) made a Observable notebook on
the Compounds of 120 5-cells. One of the cool things about this is that it also shows
the dual projection.
David Richter then made us realise that the 120 × 10 = 1200 edges of the 120 5-cells
in this compound can be obtained by uniformly extending the 1200 edges of the 120-cell and
Stellated 120-cell. If we extend these edges further, we obtain the edges of the Great
grand stellated 120-cell. This is important because it shows that the compound is isotoxal
in 4-D (and therefore, by duality, isohedral). This parallelism also applies necessarily
to their projections, so that shows that this model really is a correct projection of the
Compound of 120 5-cells. Knowing this and having Scott's vZome model made the construction
trivial. However, it should be noted that the Compound of 120 5-cells is not technically
an edge stellation of the Stellated 120-cell; as we saw above, it is instead a stellation
of the 600-cell.
When studying this compound, Nan Ma found a new regular, self-dual Compound of 720
5-cells. This was missed earlier in Coxeter's list of regular polychoron compounds! He then
found out that Peter
McMullen had discovered that regular compound in 2018! Not only that, but McMullen
also discovered five other new regular compounds, all facetings of the 120-cell: a second
Compound of 120 5-cells, a third Compound of seventy-five 16-cells, a third Compound of
seventy-five Tesseracts, and a third and fourth partially regular Compounds of twenty-five
24-cells! Finally, McMullen demonstrated that the list of regular compounds is now
complete.
That was a lot of fun!
As far as I know, this is the first physical model of this compound ever built. One has to
build something very similar during the process of building the model of the Great grand
stellated 120-cell in Figs. 6.4a and b, but there are some subtle differences that make it
very unlikely anyone has built this exact model during that process.
This ends the list of the three Zomable regular facetings of the 120-cell, and our list of
Zomable models of the regular compounds of regular polychora.
Paulo's polytope site / Next: Zomability.





















