Research Interests: Binary Pulsars!

What are binary pulsars? and... Why?

When the cores of massive stars run out of nuclear fuel they collapse catastrophically - a supernova. This compresses the core material so hard that even its atoms are destroyed, forming an incredibly dense ball of neutrons: a neutron star (henceforth NS).



Figure 1: The Crab Nebula is the remnant of a supernova that occurred in the constellation Taurus in the year 1054 AD (as seen from Earth). Credit: HST/NASA/ESA.

Pulsars are NSs that seem to pulsate in radio and gamma rays. As we discuss below, they are superb tools for studying the basic laws of the Universe. The discovery of radio pulsars in 1967, by Jocelyn Bell, demonstrated that neutron stars exist in the Universe, and that they are the end products of the lives of massive stars. This fundamental discovery resulted in a Nobel prize in Physics for A. Hewish, in 1974.

Recycled radio pulsars possess extraordinary long-term rotational stability. Timing their radio pulses, we can determine their spin periods, spin-down rate, position and proper motion with spectacular accuracy.

Because of their formation, most recycled pulsars are found in binary systems. In these cases, radio pulsar timing allows astoundingly precise measurements of the pulsar's orbital motion.

Most of the companions to recycled pulsars are also compact objects: other NSs or white dwarfs. This means that the components behave as point masses. This has a fundamental consequence: the small relativistic perturbations to the orbital motion predicted by general relativity (GR) are not mixed with any Newtonian effects caused by tides or the rotation of the companion. The precision of pulsar timing is such that some of these relativistic perturbations are measurable, in some binaries to very high precision. We thus have not only a very precise experiment, but an extremely clean one as well.

In this review, Norbert Wex and I summarise the most important GR tests with radio pulsars to date, some of these are mentioned below.

The Hulse-Taylor binary pulsar

The best known example of what can be achieved by timing binary pulsars is the study of the first binary pulsar, PSR B1913+16. The system was discovered in 1974 by Russel Hulse and Joseph Taylor using the Arecibo radio telescope. With a spin period of 59 ms, the pulsar is recycled, its companion is another NS, and the orbit is very tight (its period is 7 hours and 45 minutes) and eccentric (e = 0.61).

In this system, three relativistic effects on the orbit were measured from the pulsar timing. The first two (the advance of periastron and the Einstein delay) were used to determine the masses of the two NSs assuming GR. The third relativistic effect, a decrease of the pulsar's orbital period, matches exactly the GR prediction for the orbital decay for this system caused by the emission of gravitational waves!



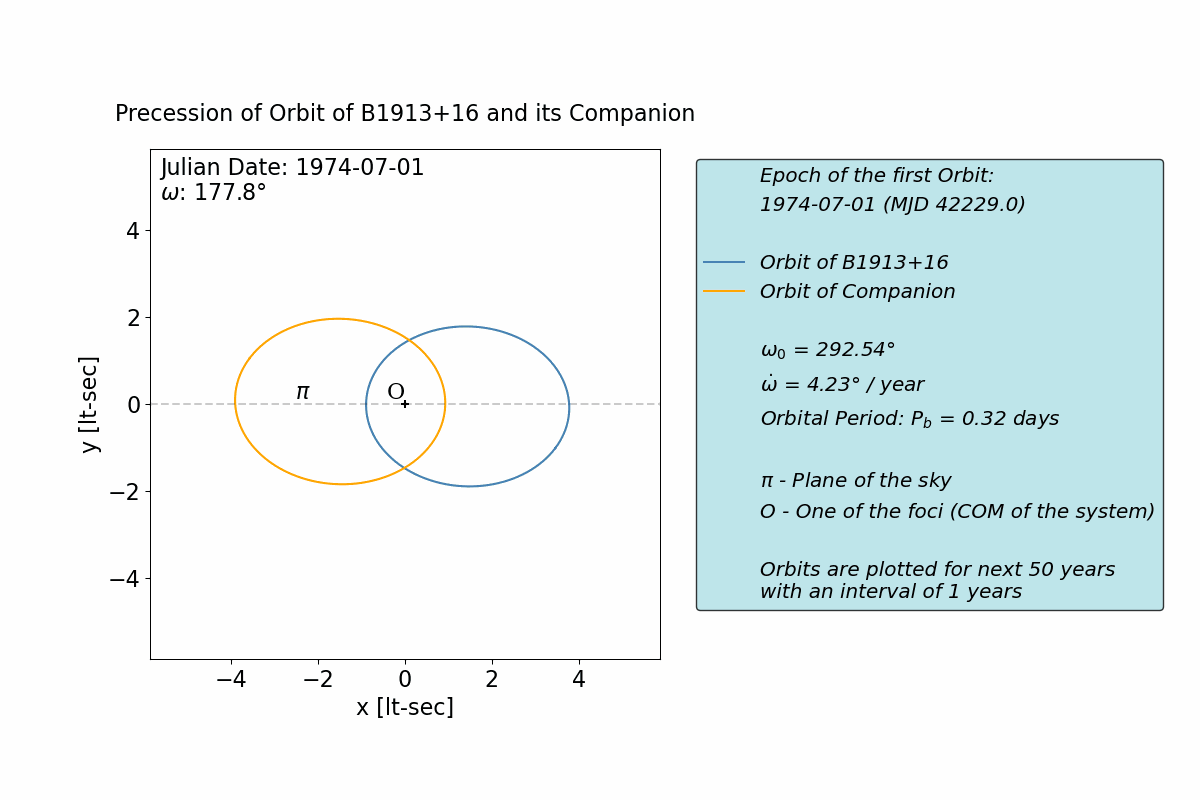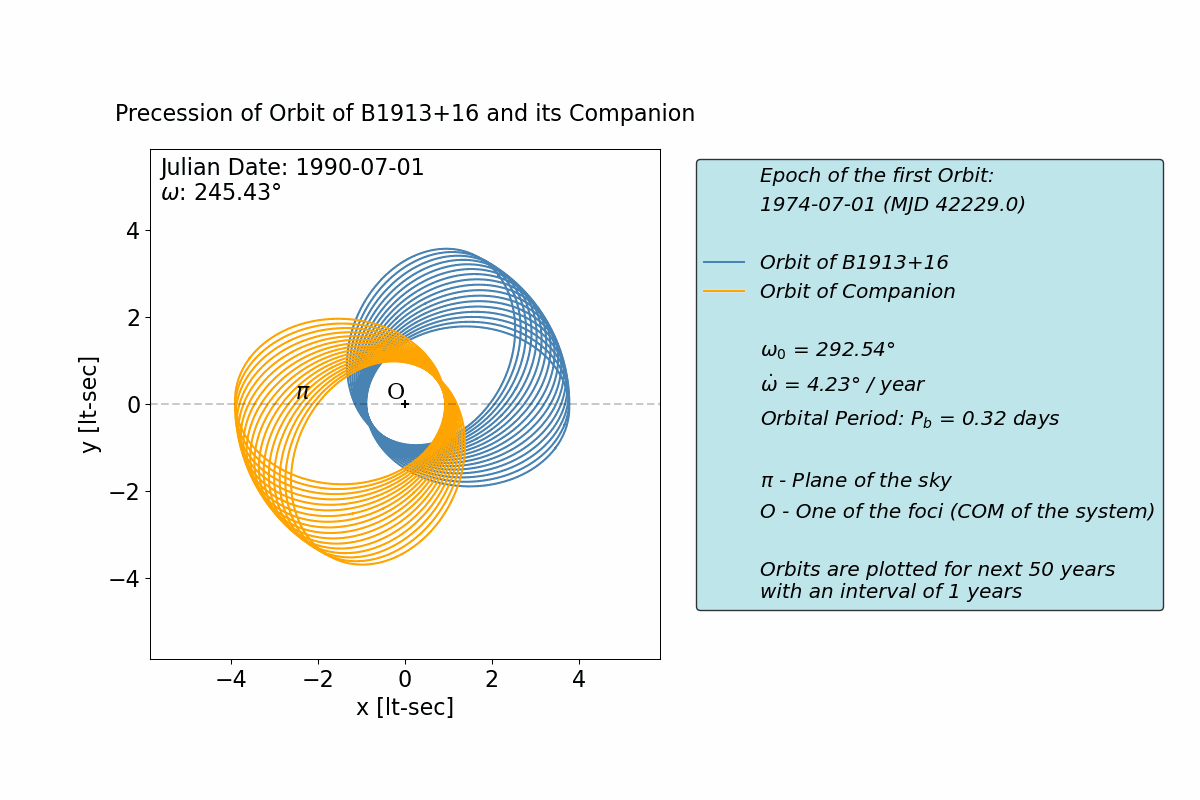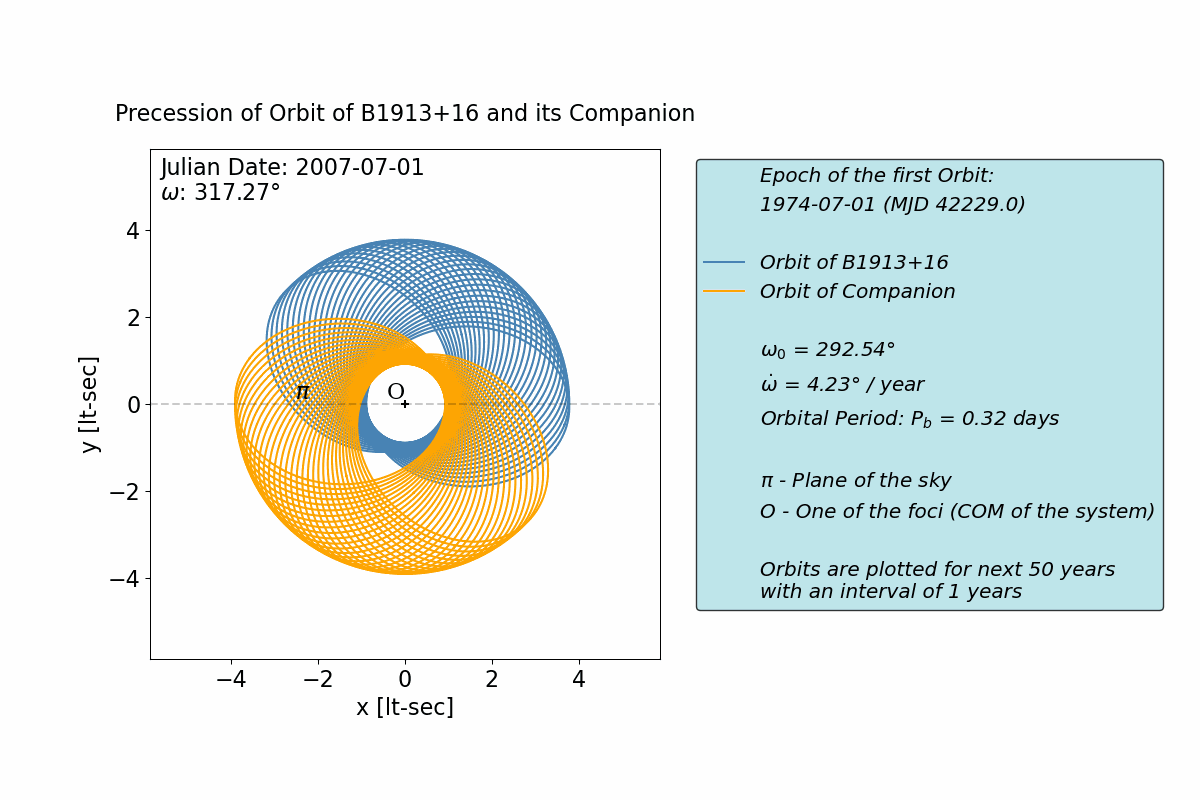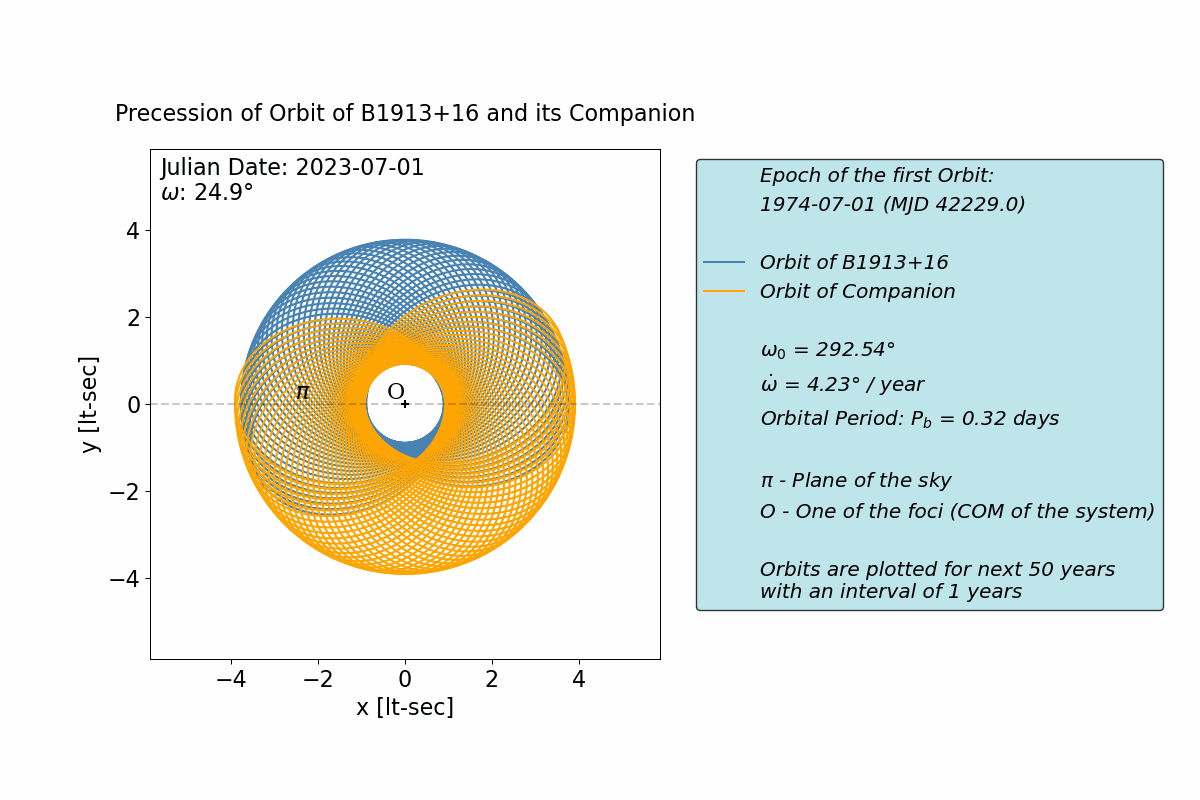
Figure 2: Orbital precession for PSR B1913+16, with the orbital orientation for the pulsar and companion plotted for the first 50 years after the discovery. The direction to the Earth is down the y axis. Credit: Paulo Freire/ Sanket Bangar.



This measurement achieved two goals that cannot be achieved by Solar System experiments: First, it confirmed the self-consistency of GR for strongly gravitating objects. But, more importantly, it confirmed experimentally the physical reality of gravitational waves (GWs) - they indeed carry energy across space as predicted by GR. This indirect detection of GWs happened decades before LIGO.

Because of the importance of their discovery, Russel Hulse and Joe Taylor were awarded the Nobel Prize in Physics in 1993.

The promise of gravitational wave astronomy

This is a supremely important discovery since GWs are not merely another relativistic effect which we can use to test GR. They are messengers that carry information across vast distances. That means that if we build an extremely sensitive GW detector, we can in principle detect faraway objects by listening to the gravitational waves they emit.

Would such a detector really hear anything at all? This is where the full importance of the discovery of PSR B1913+16 becomes clear. The orbital decay of the system implies that it will continuously lose orbital energy, with the two neutron stars inevitably merging in 330 million years. At that time they will emit a gigantic GW burst. If we observe several double NS systems in our Galaxy, there must be many, many other such systems in the Universe, with many of them merging in any particular year. This means that a sufficiently sensitive detector should certainly hear many such mergers. This process is so prevalent in the Universe that it is actually though to originate most of the heavy elements in the periodic table, like gold, platinum, lead and uranium!

It is because of this certainty that LIGO and Virgo could be built. LIGO's detection of the merger of two 30-solar mass black holes in 2015 opened up a new window on the Universe. The detection of GW170817, which was also seen throughout the electromagnetic spectrum, showed that the ground-based detectors are already sensitive enough to detect the mergers of double NS systems like PSR B1913+16.

Following the direct detection of gravitational waves with LIGO, Rainer Weiss, Barry Barish and Kip Thorne were awarded the Nobel Prize in Physics in 2017.

Research Projects

 General relativity - the current, unsurpassed description of gravity - appears to be incompatible with quantum mechanics. Furthermore, it predicts its own failure at the centres of black holes; this means that it cannot be the ultimate description of gravity.

More recently, alternative theories of gravity have been proposed to explain the rotational curves of galaxies and clusters of Galaxies without the need for Dark Matter, the accelerated expansion of the Universe without the need for Dark Energy and Cosmic Inflation (fundamental for understanding the origin of the Universe), which was driven by a still unknown force.

Is gravity really responsible for these phenomena, or are we in the presence of other forces of nature? Clearly, understanding it and testing it at all scales is important not only for understanding the laws of Nature, but also the origin, evolution and contents the Universe!

Another major puzzle arises from the unique properties of another fundamental interaction, the strong nuclear force. For densities higher than that of the atomic nucleus, the composition and behavior of nuclear matter is not known. This is a fundamental problem in nuclear physics, and in many areas of astrophysics.

Binary radio pulsars are fantastic laboratories to probe these phenomena: The extreme density of matter and the extreme gravitational fields of neutron stars mean that they probe our understanding of gravity and nuclear forces. Timing these pulsars, we can answer some detailed questions:
  1. How precisely does GR describe gravity?

  2. Can we detect phenomena beyond GR?

    3.
  3. How massive can NSs be?

  4. Are there any millisecond pulsar - black hole binaries?
Helping address some of these questions has formed the core of my research activity (see my list of refereed publications).

1. How precisely does GR describe gravity?

As mentioned above, pulsar experiments have tested GR with very high precision. Thus far, GR has passed all precise pulsar tests: GR passes the tests posed by all these systems. The last two systems are the most relativistic double NSs known in our Galaxy, even more extreme than the double pulsar (see Fig. 3). They will yield extremely precise GR tests in the near future!


Figure 3: Comparison between the sizes of the Sun, the ``classical'' pulsar - NS systems (The Hulse-Taylor system, B1913+16, and the ``Double Pulsar'' system, J0737−3039) and their tighter, more relativistic cousins, respectively J1757−1854 and J1946+2052, published in 2017 and 2018.
Credit: Norbert Wex.

2. Can we detect phenomena beyond GR?

Despite the precision of the aforementioned tests, there are other gravity theories that can pass them as well as all laboratory and Solar system tests. Most of these alternatives predict the Einstein equivalence principle (EEP) - as they must, given the very strong experimental constraints on special relativity and the weak equivalence principle.

However, and unlike GR, all viable alternatives predict the violation of the strong equivalence principle (SEP)! Therefore, the phenomena beyond GR we're most likely to observe should be consequences of SEP violation!

The main consequence of SEP violation would be a violation of the universality of free fall for strongly self-gravitation objects. This would cause, in a system where both components have very different compactness (i.e., asymmetric systems, which are unlike most pulsar - NS binaries), some potentially observable effects:
  1. The emission of dipolar gravitional waves (DGW). These can be observed as an orbital decay that is faster than the GR prediction.
  2. A polarization of the orbit of a binary system in the presence of the gravitational field of a third object, known as the Nordtvedt effect (this is a gravitational analogue of the Stark effect for neutral atoms).
One of the nice things about looking for these effects is that either we'll detect them - falsifying GR and starting a new era in physics - or we don't, falsifying any alternative gravity theories that predict them!

- What is the true nature of gravitational waves?


Over the years, we have obtained the best limits on DGW emission from our precise measurements of the orbital decay of several such asymmetric systems. These represent a profound constraint on the nature of gravitational radiation, showing that GWs are quadrupolar to a high degree of purity, as predicted by GR.
Apart from constraining DGW emission, the combination of these tests, carried out for a range of NS masses, rules out non-perturbative effects like spontaneous scalarization, which were until now possibilities in some alternative theories of gravity.

- Do NSs violate the universality of free fall?


We have looked for the Nordtvedt effect in the pulsar in a triple star system. In our measurements, the effect was not detected to within 2 parts in a million. This is the best test of the universality of free fall for strongly gravitating objects! This also represents, at the moment, the most stringent test on several wide classes of alternative theories of gravity.

3. What is the state of matter at the center of a neutron star?

One of the most important unknowns in astrophysics is the state of nuclear matter at densities higher than that of the atomic nucleus. Matter at the centre of a neutron star is precisely in this unknown state! The properties of matter under such extreme conditions can be probed from its mascrocopic behaviour, in particular the relation between density and pressure (known as the equation of state, or EOS). Each EOS predicts a unique relation between the mass of a neutron star and its radius (see Fig. 4), and also between the mass and the moment of inertia. In addition, each EOS predicts a maximum NS mass and a maximum possible spin frequency.



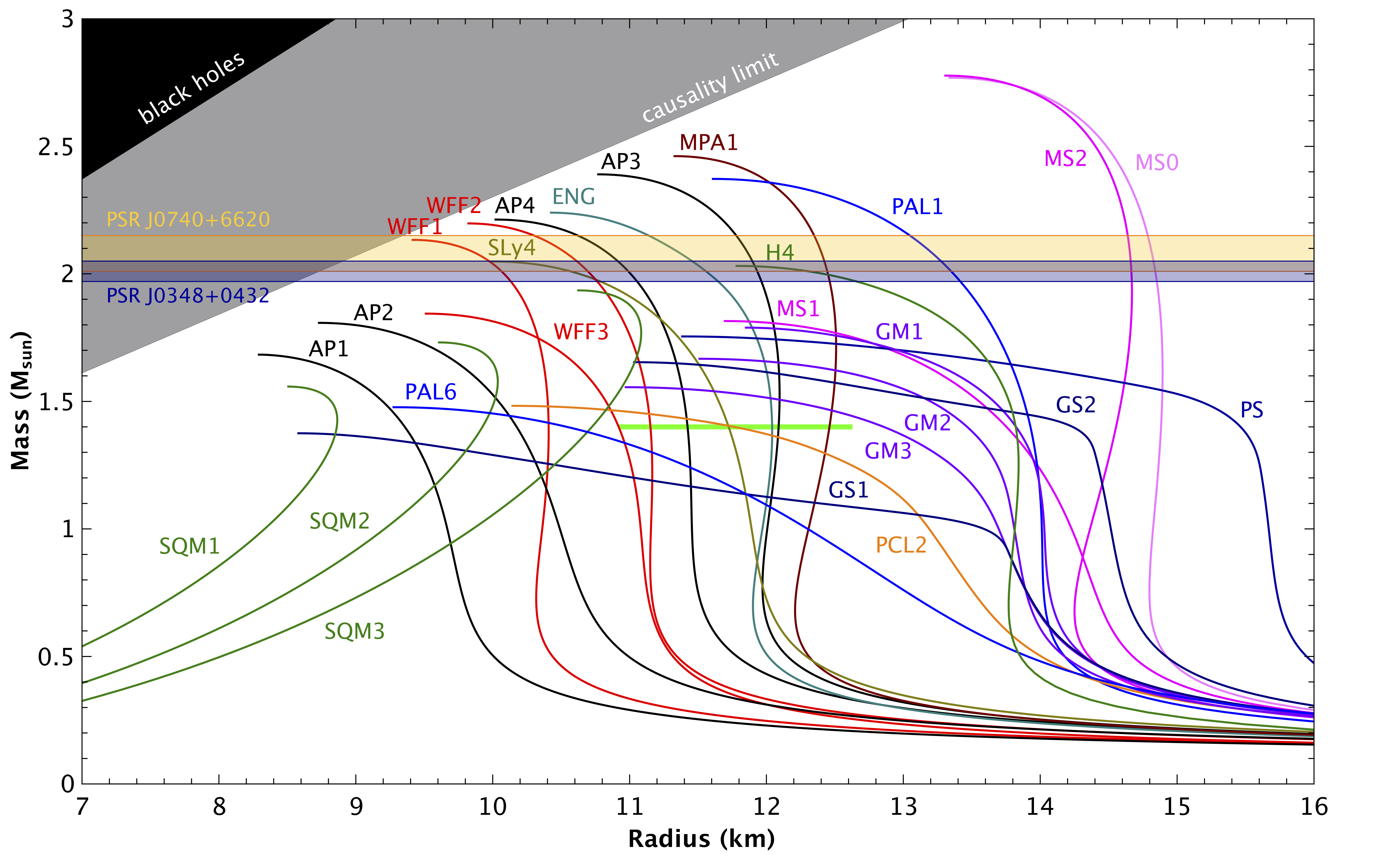
Figure 4: For each EOS (named in the figure) the relation between mass and radius for all NSs is indicated by its related curve. The 1-σ mass ranges for the two most massive pulsars known are indicated by the horizontal lines. Figure created by Norbert Wex. EOSs tabulated in Lattimer & Prakash (2001) and provided by the authors.

One of my main research topics is the measurement of NS masses. The maximum NS mass constrains the EOS: is particular EOS predicts a maximum mass smaller than the largest measured NS mass, then it is excluded. Another way to constrain the EOS is by measuring the NS moment of inertia. As mentioned above, this will be accomplished in the near future for the double pulsar system!

Look here for a review on NS masses, radii, and how they constrain the EOS.

4. Are there any millisecond pulsar - black hole binaries?

Finding a millisecond pulsar - black hole system is one of the ``holy grails'' of pulsar astronomy, as it would be a fantastic labroratory for tests of gravity theories. They are expected to form in globular clusters: In some of these, the stellar density is so high that millisecond pulsars can exchange their companions by black holes. For this reason, searching for pulsars in globular clusters is quite exciting!

A few technical innovations

Apart from this, I have also contributed with new techniques for the analysis of pulsar data:
Imprint / Privacy policy / Back to Paulo's main page.