Archimedean polytopes
Archimedean solids
Apart from the Platonic solids, there are many
other convex uniform polyhedra, which
include the two infinite classes of Prisms and Antiprisms, plus the 13 Archimedean solids. In Fig.
A.1, I show paper models of the latter, which I made in the late 1990's. Their duals are
known as the Catalan
solids, the latter include the aforementioned Rhombic dodecahedron and Rhombic
triacontahedron.

Fig. A.1: The Archimedean solids.
In the front row is the Truncated tetrahedron.
In the second row, from left to right, is the Cuboctahedron, the Truncated octahedron, the Truncated cube, the
Rhombicuboctahedron, the Truncated
cuboctahedron (also known as the Omnitruncated cube or octahedron) and the Snub cube.
In the back row, from left to right, is the Icosidodecahedron, the Truncated icosahedron, the
Truncated
dodecahedron, the Rhombicosidodecahedron,
the Truncated
icosidodecahedron (also known as the Omnitruncated dodecahedron/icosahedron) and the
Snub
dodecahedron.
The front row has objects with Tetrahedral symmetry. The
second row has objects with Octahedral symmetry. The last
row has objects with Icosahedral symmetry.
However, the Snub cube and the Snub dodecahedron lack mirror symmetry - they are different
from their mirror images. Their symmetries are, respectively, the chiral
ochahedral and the chiral icosahedral symmetries.
These solids are illustrative examples of different types of the Wythoff construction. This
construction is based on the idea of symmetry as a reflection. In an object with, for
instance, bilateral symmetry, the left and right sides are identical, but appear as if
reflected in a mirror. Thus, in this case, we could start with the right side of the
object and obtain the left side by reflecting it in a mirror, generating the whole
symmetric object.
Thus, any highly symmetric figure - like all regular polytopes - can be buit from
reflections in a particular set of mirrors: a kaleidoscope. For symmetric
polyhedra, these mirrors are the edges of a spherical Schwarz triangle (which can tile of a spherical
surface), which are the fundamental domain of a 3-D
point symmetry group.
In Fig. A.1, we meet several types of Wythoff construction. The regular polyhedra and
their rectifications (the latter are shown in the first column on the left in Fig. A.1)
share the same type of Wythoff construction: Their vertices are reflections of one of the
three vertices of the Schwarz triangle. This means that for each pair of dual regular
polyhedra (i.e., for each type of symmetry) there is only one rectified form. A
consequence of this type of Wythoff construction is that all regular polyhedra and
rectifications are isotoxal, i.e., all edges are identical.
The difference between the rectification and the regular polyhedra with the same
symmetry is that the vertex being reflected is at the right angle of the Schwarz
triangle, thus at the intersection of two perpendicular mirrors. Thus, any edge
touching that vertex is reflected by those mirrors, generating a rectangular vertex
figure. This implies that all edges, apart from being identical, separate two types of
faces. Furthermore, as we've seen already in the polyhedron page, the central symmetry of these vertex
figures implies that these polyhedra have equatorial Polygons.
Another type of Wythoffian construnction is the truncation. Here, the vertices of a
regular polyhedron are cut, as in a rectification, but not so deeply that the cuts touch
each other, instead leaving a length of edge between the previous faces that is identical
to the edges of the cuts. For each symmetry, there are three truncated forms (columns 2, 3
and 4 in Fig. 1), where the vertices result from reflecting points in the edges of the
Schwarz triangle, i.e., located in one of the mirrors. All their vertex figures will,
because of this, have bilateral symmetry.
A third type of Wythoffian construnction is the omnitruncation (column 5 in Fig. A.1),
where the vertices result from reflecting points inside the Schwarz triangle. There
is only one such polyhedron for each type of symmetry. This means that in a polyhedron
generated this way, the number of vertices will be, for a particular symmetry, the same as
the number of Schwarz triangles that tile the spherical surface, which is the order of the
associated symmetry group. The Omnitruncated octahedron/cube has 48 vertices and the
Omnitruncated icosahedron/dodecahedron has 120 vertices (more about the Omnitruncated
tetrahedron next). The vertex figure will be close to the dual of the Schwarz triangle.
Finally, there is a particular type of transformation that produces one snub form for each
symmetry. This works by alternate deletion of Schwarz triangles.
***
In Fig. A.1 we only show one polyhedron derived from the Tetrahedral symmetry, the
Truncated tetrahedron. The reason for this is that when we
apply the different Wythoff constructions to the Tetrahedral symmetry, the resulting
forms are, with the exception of the Truncated tetrahedron, redundant with polyhedra
derived from other symmetries. As mentioned in the polyhedron page, the Rectified tetrahedron is the Octahedron.
In addition, the Rhombitetrahedron is the Cuboctahedron; the Omnitruncated tetrahedron is
the Truncated octahedron (which, like all omnitruncated simplexes, is a permutohedron, all of which can
fill the Euclidean space they are embedded in); this has 24 vertices, which is the order
of the Tetrahedral symmetry.The Snub tetrahedron is the Icosahedron.
Therefore, many polyhedra have multiple Wythoff constructions, i.e., they can be derived
from different symmetries. The overlap in forms between the Tetrahedral and Octahedral
symmetries is not surprising as the Tetrahedron is the demi-cube (the 3-dimensional
version of a Demihypercube, obtained by
alternate deletion of vertices of Hypercubes).

Fig. A.2: The Platonic (three polyhedra on the left) and Archimedean (three polyhedra on
the right) solids with Tetrahedral and Octahedral symmetries that can be built with the
Zometool. The polyhedra in green can be derived from the Tetrahedral symmetry.
Finally, one word on which of these polyhedra are Zomable:
- All polyhedra derived from the Tetrahedral symmetry are Zomable, all with green struts
(see Fig. A.2) except the Icosahedron, which is Zomable with blue struts.
- All polyhedra derived from the Icosahedral symmetry are Zomable, all with blue struts,
except the Snub icosahedron, which is not Zomable.
- However, several polyhedra derived from the Octahedral symmetry - the Truncated cube,
the Rhombicuboctahedron, the Truncated cuboctahedron and the Snub cube - are not Zomable.
Except for the latter, the reason is that they have Octagons as faces or edge sections, which
cannot be displayed in the Zometool system.
Indeed, of the polyhedra derived from Octahedral symmetry, only those that can also be
derived from Tetrahedral symmetry - and the Cube - are Zomable (see Fig. A.2)! This will
have some interesting implications below.
Just like we can apply the concept of the Wythoff construction to polyhedra, we can also
apply it to the regular tilings of 2-D Euclidean space, which are the flat analogs of
the polyhedral surface. Doing this, we obtain all the Archimedean tilings of 2-D Euclidean
space, with the exception of the elongated triangular
tiling, which is non-Wythoffian. These tilings are of practical interest for fields
like architecture, design and engineering.
Finally, it should be noted that if we lift the condition of convexity, several other
types of Uniform polyhedra become possible: an infinite family of Star prisms and
antiprisms, and a finite family of ``Star Archimedeans'', which include the regular star
polyhedra (Fig. 3.5a), their rectifications (Fig. 3.6a) and the ditrigonal polyhedra (Fig.
3.7) as special cases. Most of these are obtained by the Wythoff construction acting on Schwarz triangles with non-integer
indices, with one exception, the Great
dirhombicosidodecahedron.
Archimedean polychora
The uniform
convex polychora include, in addition to the regular convex polychora, several types of 4-D
prisms and 47 non-prismatic polychora. By analogy with the Archimedean solids, I will
refer to the latter as the "Archimedean" polychora.
Like the Archimedean solids, these Archimedean polychora illustrate different types of
Wythoff construction. The mirrors of the underlying Kaleidoscope are the faces of
spherical Goursat
tetrahedra (which tile the 3-sphere), which are the fundamental
domains of a 4-D point symmetry
group. All but one of the 47 Archimedean polychora can be built in this way; the
exception is discussed below.
Rectifications:
As for the polyhedra, the regular polychora and their rectifications also share the same
type of Wythoff construction: their vertices are reflections of one of the four
vertices of the Goursat tetrahedron. For most symmetries, this implies that for each dual
pair of dual regular polychora, there are two rectified polychora. As in the case
of the rectifications among the Archimedean solids, all of these forms are isotoxal.
Also, as for the regular counterparts, all rectifications are Zomable. In the page on the
regular convex polychora we have already
seen models of the Rectified 5-cell (Fig. 5.3), the Rectified 16-cell (which is regular,
the 24-cell, Figs. 5.5a and b) and the Rectified 24-cell (Fig. 5.6). Below we show the
rectifications of the three remaining convex regular polychora.
We first present two projections of the Rectified tesseract. The 8
Cubes of the tesseract were rectified, becoming Cuboctahedra. Under the 16 vertices of the
Tesseract new Tetrahedra appeared. Each edge is shared by one Tetrahedron and two
Cuboctahedra.
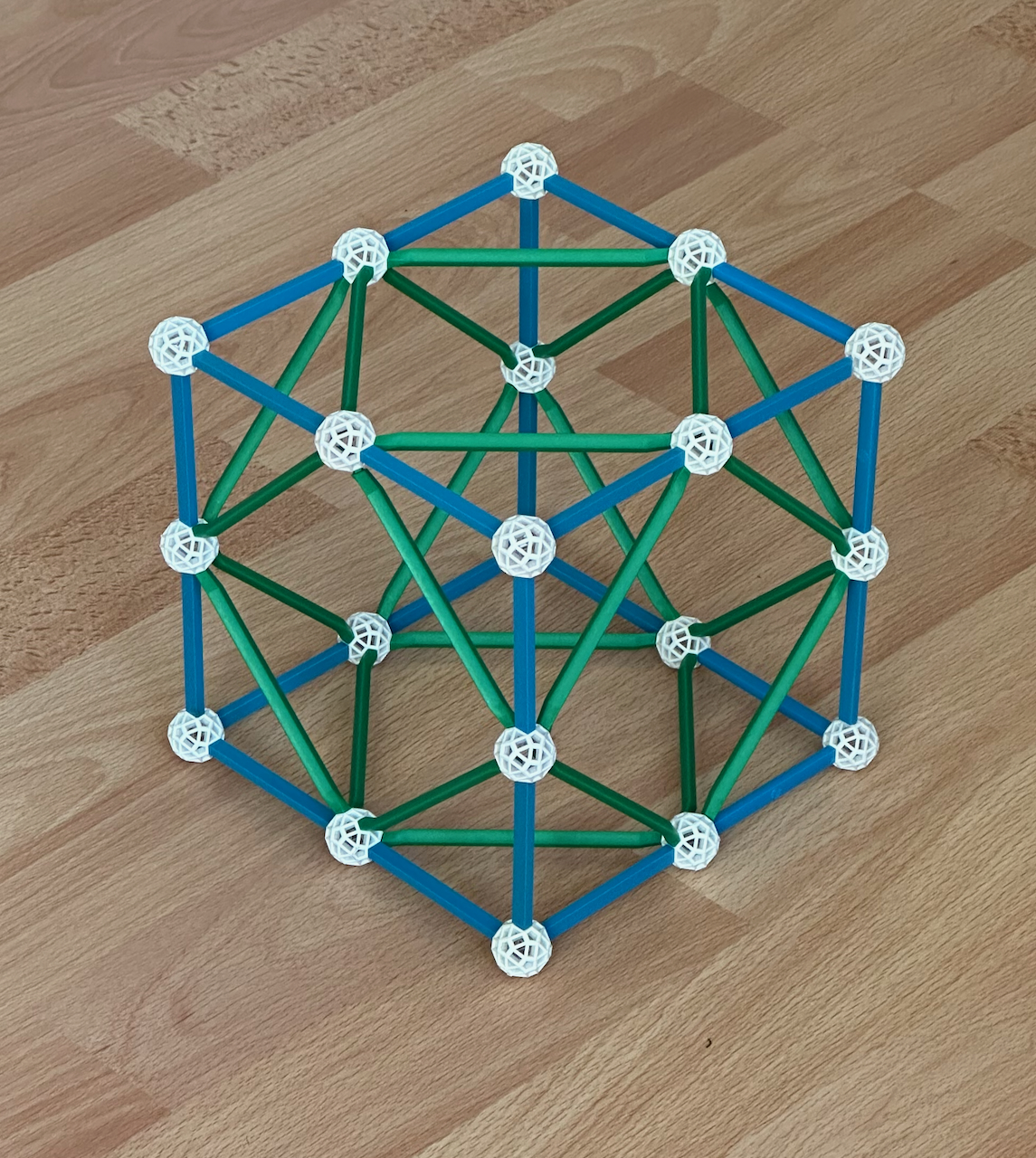
Fig. A.3a: Projection of the Rectified tesseract, centred on a Cuboctahedral cell. This
projection is the rectification of the cell-first projection of the Tesseract in Fig. 5.4.

Fig. A.3b: Projection of the Rectified tesseract centered on the Tetrahedral cells(*).
This projection is the rectification of the vertex-first projection of the Tesseract in
Fig. 5.4.
The model in Fig. A.3b represents the rectification of the vertex-first projection of the
Tesseract in Fig. 5.4. The Yellow rhombuses of that projection, which are represented by
yellow struts, get rectified into Yellow rectangles, which have green and blue struts (see
Fig. 2.5a). Unlike the projection of the Rectified tesseract in Fig. A.3a, but similarly
to what happens with the vertex-first projection of the Tesseract, the central element
lacks central symmetry, which means that the "near" and "far" sides of the projection do
not coincide (hence the asterisks). For that reason, the opposite Tetrahedra supperpose in
a Stella Octangula configuration. This means that this model represents, in fact, two
different projections, each with one of the two Tetrahedra at the center closer to us.
In the page on the regular convex polychora,
we have seen two semi-regular convex
polychora (which, apart from being Uniform, have only regular cells, in these cases of
two distinct types): the Rectified 5-cell and the Snub 24-cell. There is a third and last
semi-regular convex polychoron, the Rectified 600-cell. Like the
Rectified 5-cell, this results from rectifying a regular convex polychoron with
Tetrahedral cells: the 600 Tetrahedra of the 600-cell are replaced by their
rectifications, Octahedra. Under each of the 120 vertices of the 600-cell new Icosahedra
(the vertex polyhedron of the 600-cell) appeared; all their projections were already
visible in the model of the 600-cell in Fig. 5.10. Each edge is shared by one Icosahedron
and two Octahedra. Its Icosahedral projection, centered on an Icosahedral cell, is shown
in Fig. A.4.
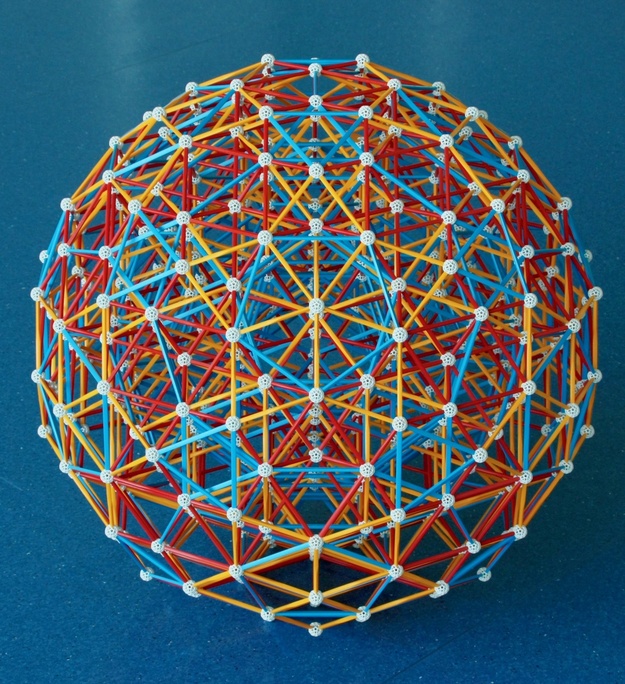
Fig. A.4: The Icosahedral projection of the Rectified 600-cell. This projection is
the rectification of the Icosahedral projection of the 600-cell.
How to build: After studying the perspective-flattened Icosahedra in the model of
the 600-cell, have a look at the Eusebeia page on the Rectified 600 cell.
Also recommended is David Richter's page on
the Rectified 600-cell.
Also, as for the other semi-regular polychora, all faces are Triangular. In all
rectifications of regular polychora with Tetrahedral cells the vertex polyhedron is a
prism, in this case it is Pentagonal. Since all faces are Triangular, these prisms are
necessarily edge sections located under each vertex.
If you pay close attention to the model, you will be able to see several Archimedean
solids in blue, starting with Icosidodecahedra - the rectifications of the Icosahedral
sections of the 600-cell. These polyhedra are edge sections of the Rectified 600-cell.
Because they have Icosahedral symmetry, they appear ``under'' the Icosahedral cells in 4-D
space, around the central cells in this projection. Studying the model further, you will
be able to see many flattened versions of those.
All these sections are the cells of 14 non-convex polychora, all of them edge facetings of
the Rectified 600-cell and therefore represented by the same model. One of them is the
Rectified icosahedral 120-cell, which has Great dodecahedra and Icosidodecahedra as cells
(for more about the Icosahedral 120-cell, see the page on regular star polychora). These 14 edge-facetings
are a sub-group of the 60 facetings of the Rectified 600-cell
that are uniform, non-convex polychora.

Fig. A.5: The icosidodecahedral cell-first projection of the Rectified 120-cell.
How to build: Study the perspective-flattened Dodecahedra in the model of the
120-cell. Make models of Icosidodecahedra with similar flattening. Then have a look at the
Eusebeia page on the
Rectified 120 cell.
Picture taken by Jason Wu.
Finally, in Fig. A.5 we show the Icosahedral projection of the Rectified 120-cell. The
Dodecahedra of the 120-cell are here replaced by their rectifications, Icosidodecahedra.
Under each of the 600 vertices of the 120-cell, a Tetrahedron (the vertex figure of the
120-cell) appeared. Each edge is shared by a Tetrahedron and two Icosidodecahedra. This
Icosahedral projection is necessarily centred on an Icosidodecahedral cell.
Truncations:
We now show a few examples of truncations. The first example is the Truncated
16-cell. In this polychoron, we cut the 8 vertices of the 16-cell, which produces 8
new polyhedral cells that are identical to the vertex figure of the 16-cell, Octahedra.
However, the 16 Tetrahedral cells of the 16-cell were not rectified to Octahedra (as in
the Rectified 16-cell, also known as the 24-cell), but instead truncated, becoming
Truncated tetrahedra. All vertices are still identical, so that this new polychoron is
still uniform.
However, like the Truncated tetrahedron, this polychoron is no longer isotoxal: we have
now not only different types of cells and faces, but also different types of edges. The
same applies to the following polychora.
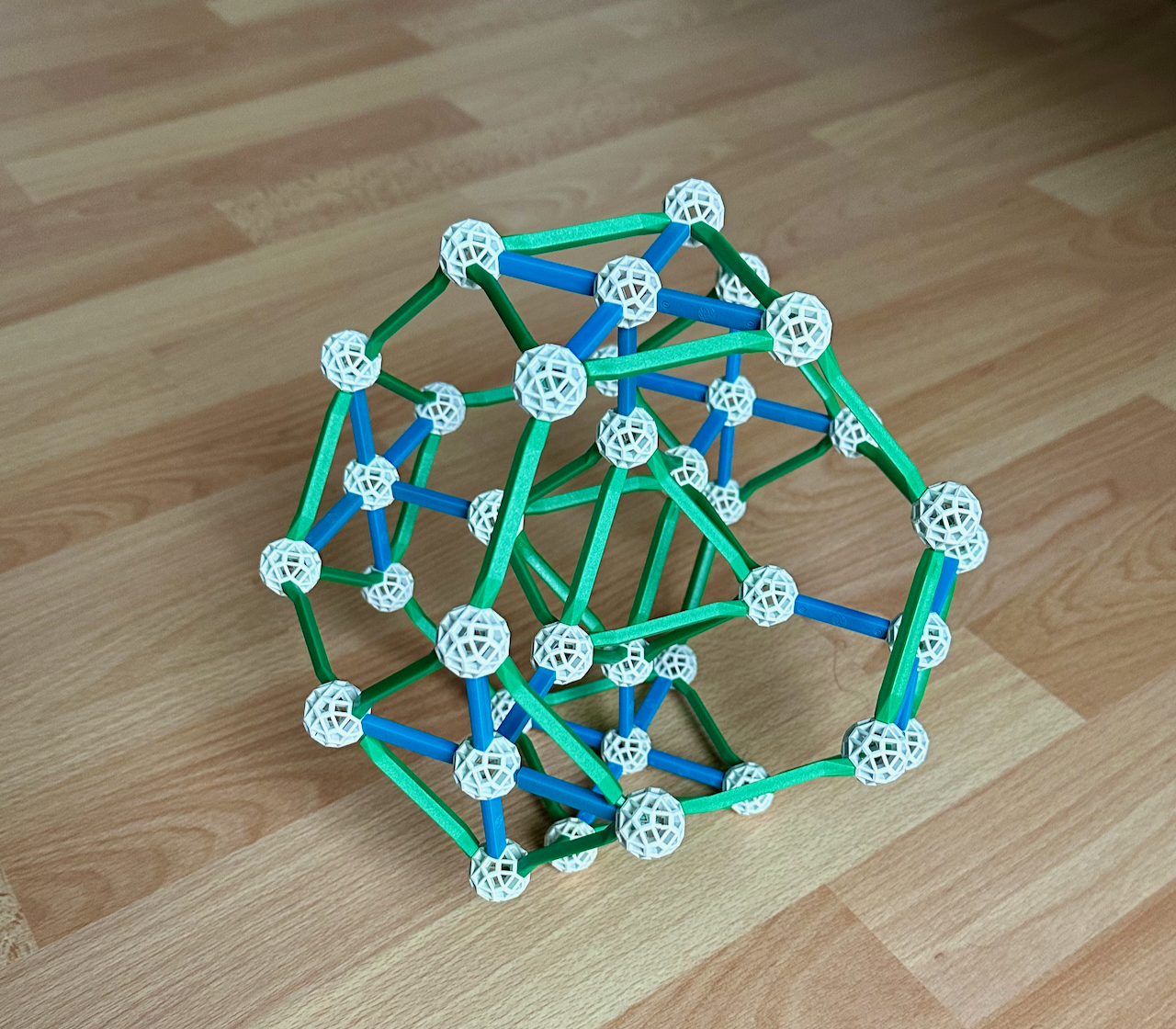
Fig. A.6a: The truncated 16-cell. This projection, centred on an Octahedron, is the
truncation of the vertex-first projection of the 16-cell in Fig. 5.4.
How to Build: See the Eusebeia page on the Truncated 16-cell.

Fig. A.6b: The truncated 16-cell*. This projection, centred on two Truncated tetrahedra,
is the truncation of the cell-first projection of the 16-cell in Fig. 5.4. For the same
reason, it can represent two truncated 16-cells, depending on which Truncated tetrahedron
is closest to us.
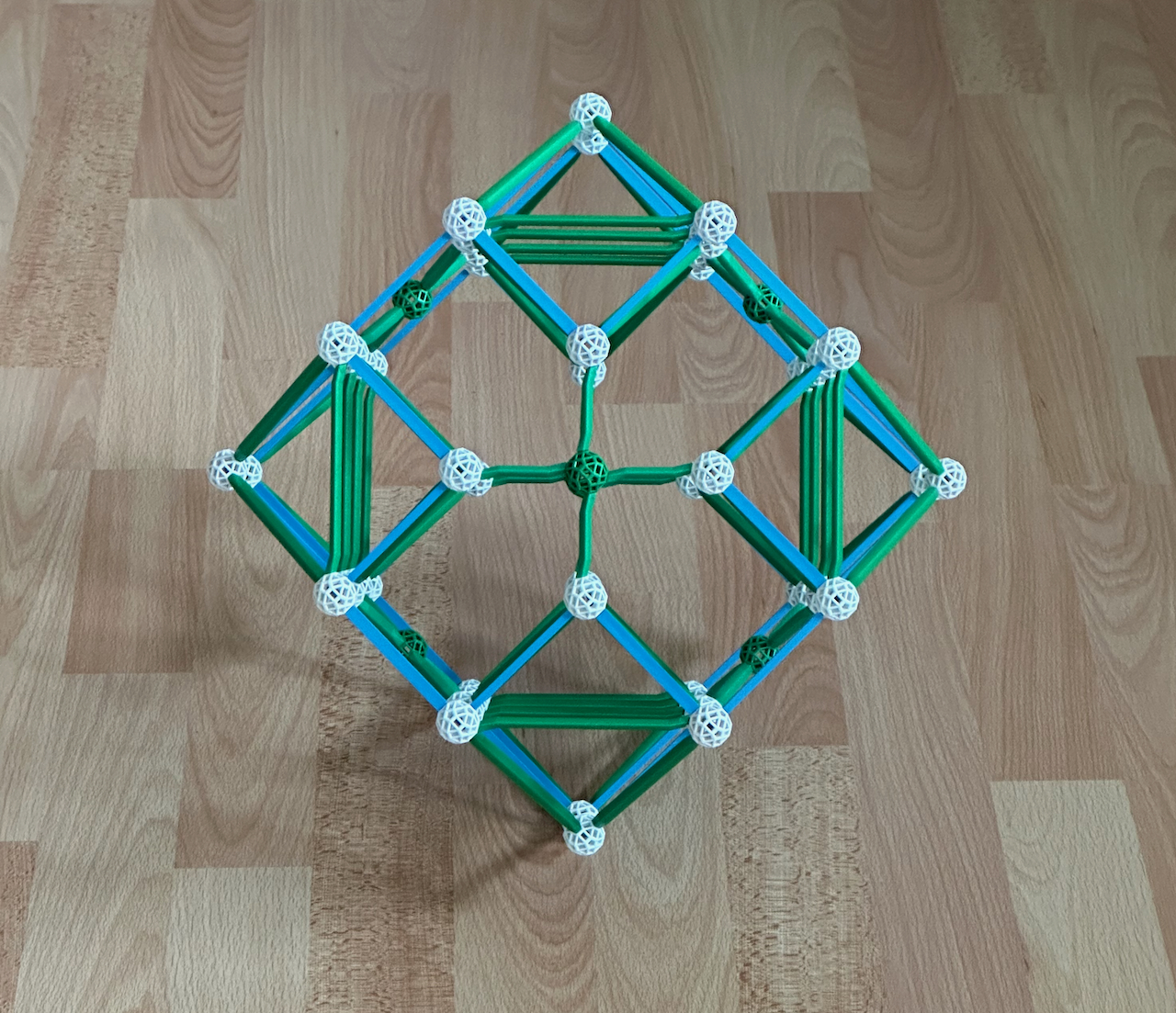
Fig. A.6c: A different perspective of the last model.
We cannot represent the Truncated tesseract, because it has Truncated cubes as cells.
These have Octagonal faces, which as mentioned above cannot be represented properly in the
Zometool system. However, we can represent the Bi-
truncated tesseract, which is also the Bi-truncated 16-cell. This is a special type of
truncation, where the Tetrahedral and Cubic cells of the two dual polychora are both bitruncated, resulting
respectively in Truncated tetrahedra and Truncated octahedra.
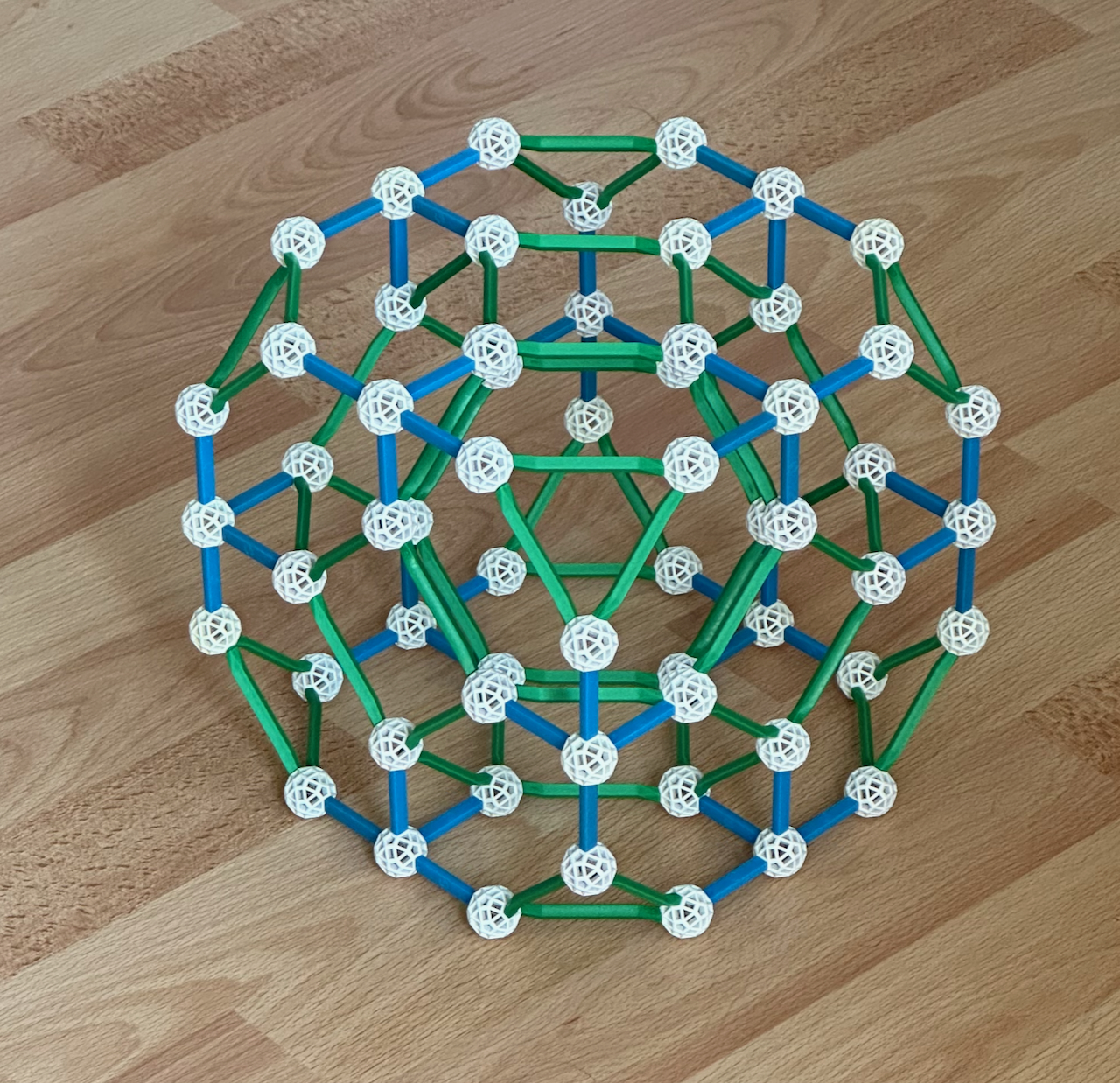
Fig. A.7a: The bitruncated Tesseract/16-cell. The projection is centred on a Truncated
octahedron.
How to Build: See the Eusebeia page on the Bitruncated
tesseract.
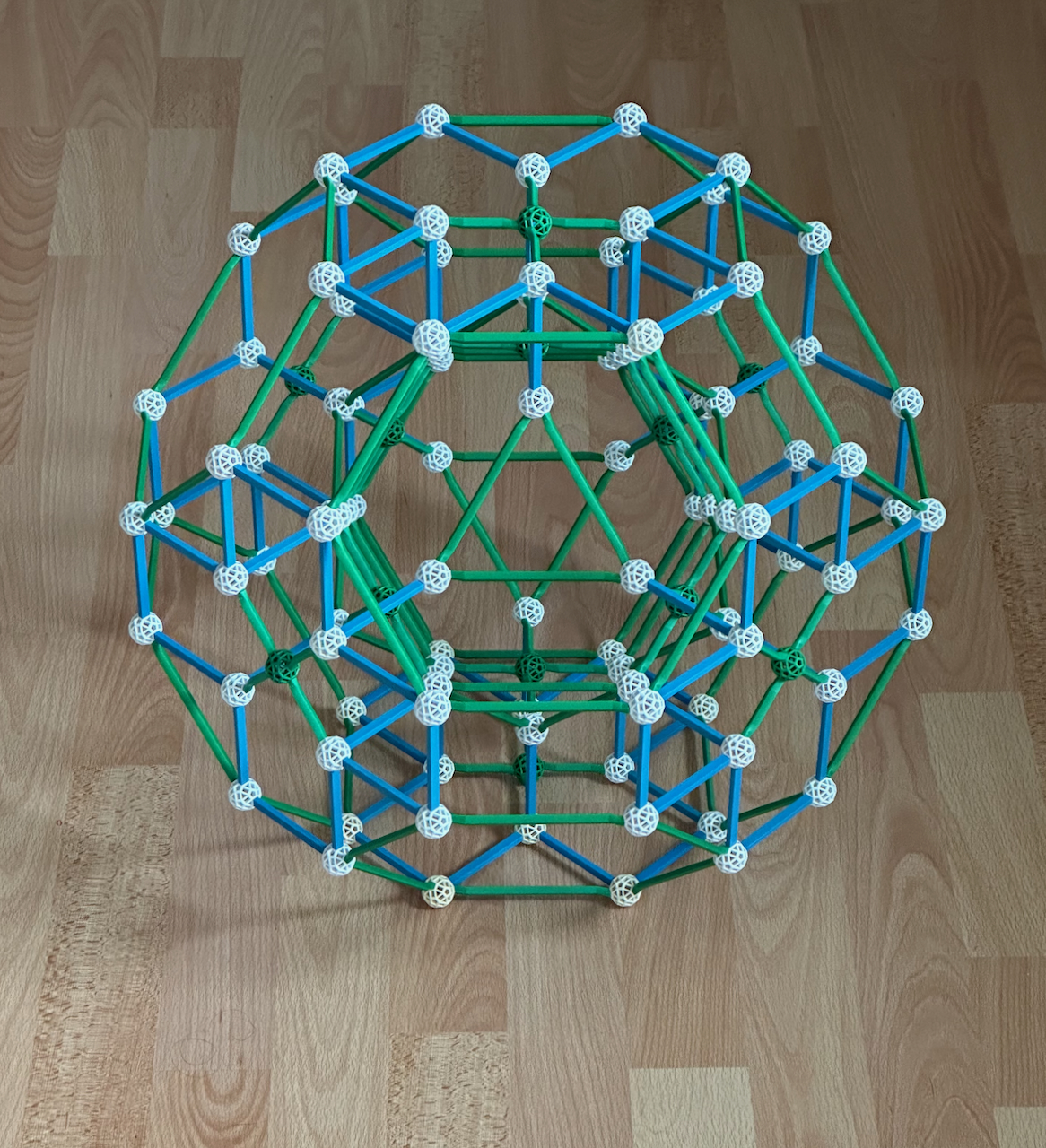
Fig. A.7b: The bitruncated Tesseract/16-cell*. This projection is centred on two Truncated
tetrahedra. It can represent two bitruncated Tesseract/16-cells, depending on which
Truncated tetrahedron is closest to us.

Fig. A.7c: A different perspective of the last model.
Another important example is the Truncated
24-cell. As in the Rectified 24-cell, we cut the 24 vertices of the 24-cell, which
produces 24 new Cubic cells. However, the 24 Octahedral cells of the 24-cell are not
rectified, but truncated into Truncated octahedra.
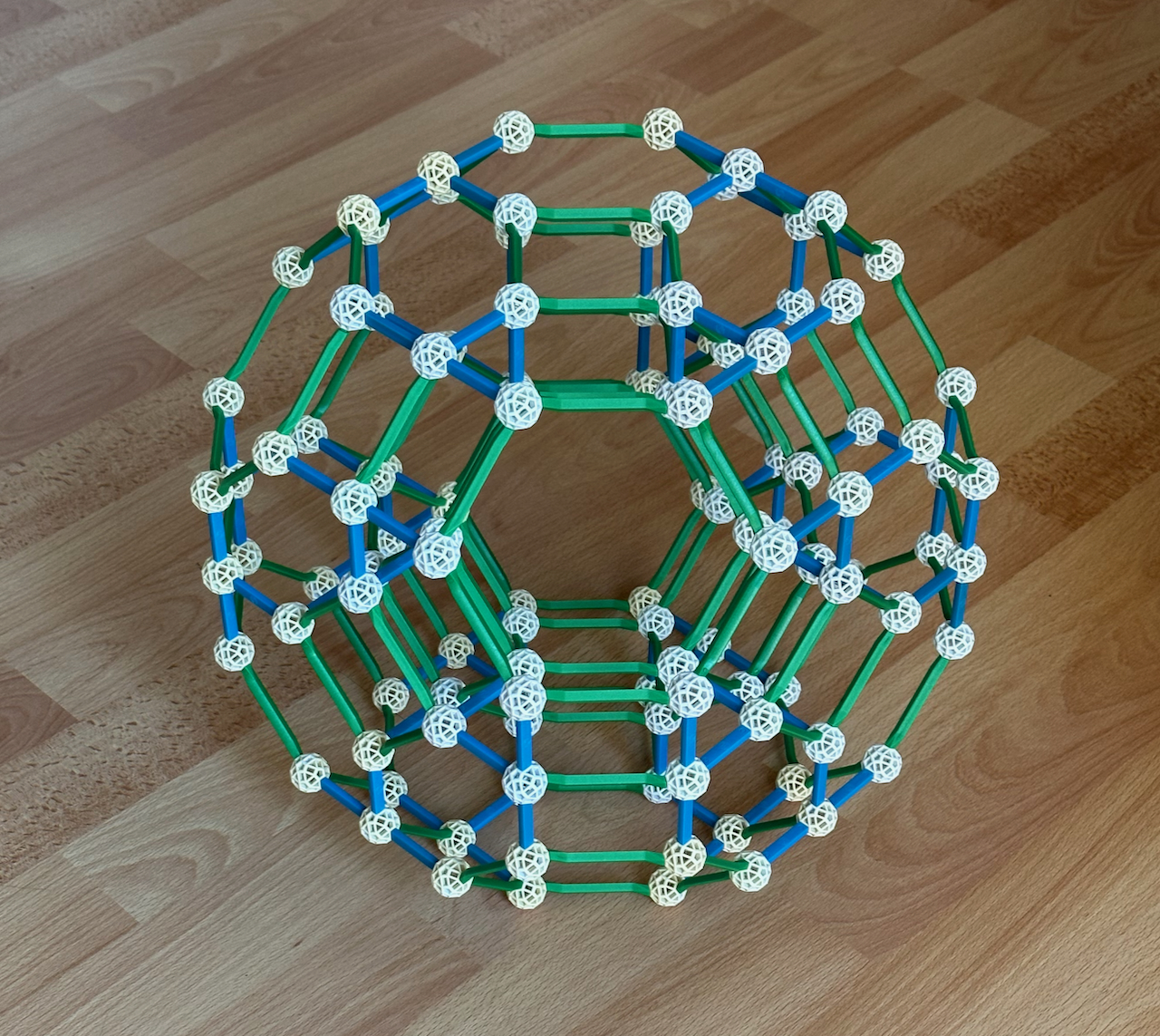
Fig. A.8: The truncated 24-cell. The projection, centred on a Truncated octahedron, is the
truncation of the cell-first projection of the 24-cell.
How to Build: See the Eusebeia page on the Truncated 24-cell.
For this particular projection, see the part on the "cantitrucated 16-cell".
Finally, we show the Truncated
600-cell, a model of its Icosahedral projection is shown below. It is similar to the
Rectified 600-cell above, and it also has 120 Icosahedral cells. However, instead of 600
Octahedral cells, it has 600 Truncated tetrahedral cells.

Fig. A.9a: The Truncated 600-cell, here seen along one of its 5-fold symmetry axes.

Fig. A.9b: The same model as above, seen here from a 3-fold symmetry axis.
How to Build: See the Eusebeia page on the Truncated 600-cell
As for the Archimedean polyhedra, the vertices of truncated (or bi-truncated) polychora
are reflections of points located on edges, this time on one of the six edges of the
Goursat tetrahedron.
Other constructions:
A few other types of Wythoffian construnctions can also generate uniform polychora while
reflecting points located in the four faces of the Goursat tetrahedron. An example of this
with the symmetry of the 600-cell is the
cantitruncated 600-cell (this page also shows how to make all Wythoffian constructions
of polychora using vZome).
The most complex type of Wythoffian construction is the ommnitruncation, which reflects a
vertex inside the Goursat tetrahedron. This means that in a polychoron generated
this way, the number of vertices will be, for a particular symmetry, the same as the
number of Goursat tetrahedra that tile the 3-sphere, which is the order of the associated
symmetry group. There are two Zomable examples. The first is the Omnitruncated
5-cell (see vZome model), which is the 4-dimensional permutohedron and is
therefore one of the rare uniform polychora that can fill space. Furthermore, this
polychoron is twice as symmetric as the 5-cell or the rectified 5-cell, having the full
extended pentachoric symmetry, which includes central symmetry. This property is shared by
all permutohedra at all dimensions.
This property is also shared the other omnitruncation of a self-dual polychoron, the
Omnitruncated 24-cell: the reason why it has twice the symmetry of the 24-cell is that
both dual sets of axes pass through identical sets of cells (the axes with Octahedral
symmetry go though Truncated cuboctahedra), being therefore treated identically: in the
24-cell, one set of axes with Octahedral goes through cells, the other through vertices.
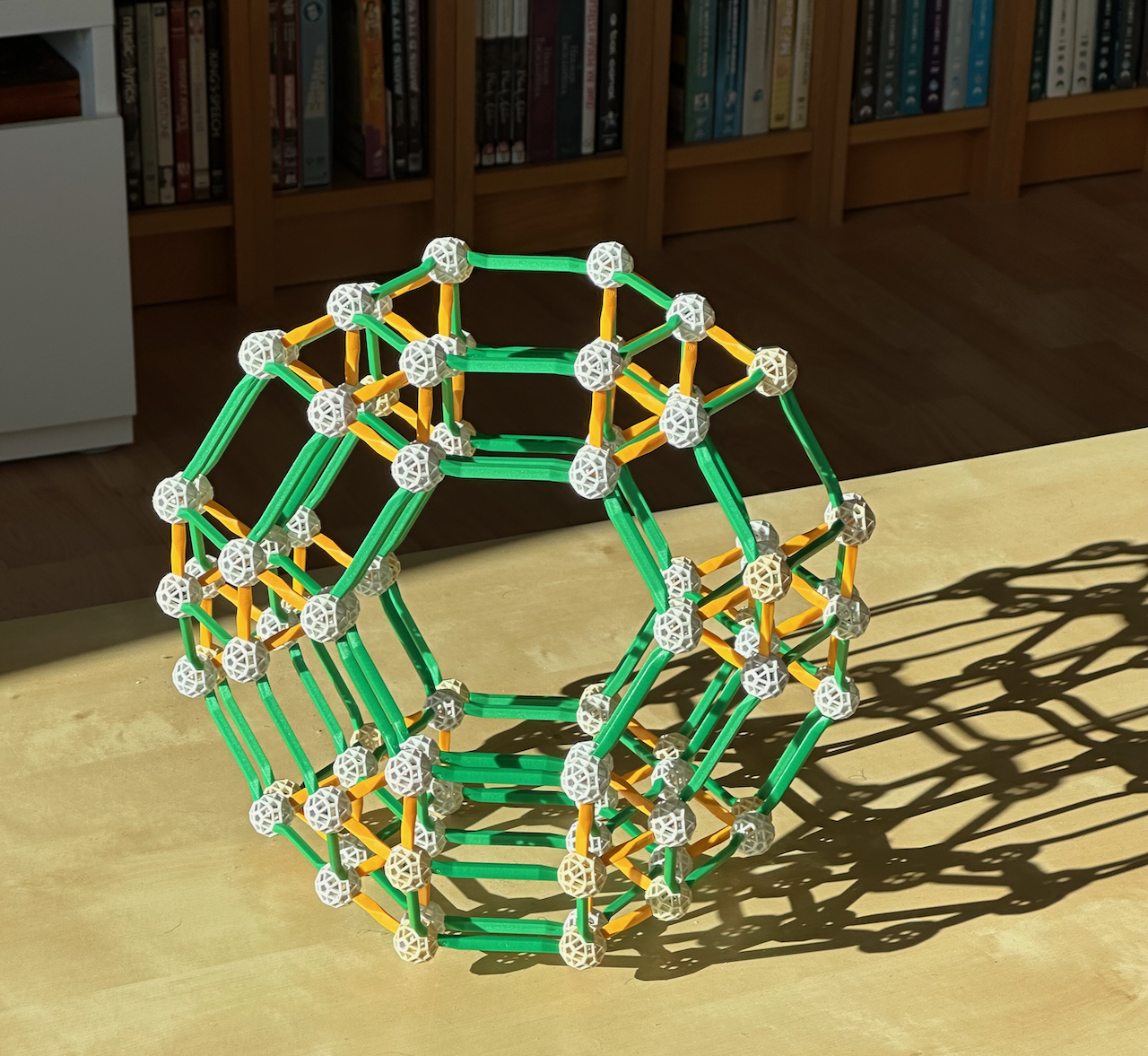
Fig. A.10: The Omnitruncated 5-cell(*). The projection is centred on a Truncated octahedron.
How to Build: See the Eusebeia page on the Omnitruncated 5-cell.
However, the Omniruncated 24-cell is not Zomable. The second Zomable omnituncation is the
Omnitruncated 120-cell. This polychoron has 14400 vertices, the number of symmetries
of any object with full Hexacosichoric symmetry. Its Icosahedral projection is
Zomable, but it dwarfs the complexity of the model in Figs. A.9a and b (see video).
***
The discussion above implies that, for each 4-dimensional point symmetry, there are in
principle 4 uniform polychora that result from reflecting the 4 vertices of the Goursat
tetrahedron (2 regular and their 2 rectifications), six from the 6 edges of the Goursat
tetrahedron (which include the truncations and bitruncations mentioned above), four from
the 4 faces of the Goursat tetrahedron, and the Omnitruncated form that results from
reflecting a point inside the Goursat tetrahedron. We thus come to a total of 15 uniform
polychora for each type of polychoral symmetry. However, for the symmetries of self-dual
forms, where a polychoron and its dual are identical (the same applying to their
rectifications and other forms), we have a total of 9 uniform
polychora.
Thus, the number of Archimedean polychora should be:
- 9 (symmetry of the 5-cell) +
- 9 (symmetry of the 24-cell) +
- 15 (symmetry of the 16-cell) +
- 15 (symmetry of the 600-cell) +
- The semi-regular Snub 24-cell (Fig. 5.8), a special
form derived from the symmetries of the 16-cell and 24-cell, +
- The grand antiprism (see next) = 50.
However, as mentioned above, there are only 47. The reason is that three objects are being
counted twice: the 24-cell is also the rectification of the 16-cell, the Rectified 24-cell
is also the "cantellated 16-cell" (see caption of Fig. 5.6) and the Truncated 24-cell is
also the "cantitruncated 16-cell" (see caption of Fig. A.8). This situation is analogous
to the cells of the 24-cell, Octahedra, which can be derived from the Tetrahedral and
Octahedral symmetries. The number of Archimedean polychora derived from the symmetries of
the 16-cell and 24-cell is thus 15 + 9 + 1 − 3 = 22.
The Grand antiprism:
The Grand
antiprism was the last Archimedean polychoron to be discovered (in 1964 by John H.
Conway and Michael Guy), who also proved that this is really the last one. This is an
anomalous uniform polychoron in the sense that, unlike all other polychora discussed
above, it has no
Wythoffian construction.
The Grand antiprism and the aforementioned Snub 24-cell are diminished
600-cells; this means that their vertices and edges are subsets of the vertices and
edges of the 600-cell. Since the 600-cell is Zomable, this implies that the Grand
antiprism and the Snub 24-cell must be Zomable as well! A Zomable projection of the Snub
24-cell appears in Fig. 5.8. Below I show two Zomable projections of the Grand antiprism,
both are derived by removing selected struts and vertices from the Icosahedral projection
of the 600-cell.

Fig. A.11a: A projection of the Grand antiprism.
Picture by Aris Noutsos. Linked picture by Jason Wu.
How to Build: You can see a vZome model of this projection (together with other
3-D projections) at the start of this page.
When you study the model of the 600-cell in detail, you realize that there are six red
axes going through the model (which are Decagons flattened into lines by the projection)
and that, perpendicular to them, there are six blue Decagons defining an outer blue
Icosidodecahedron. All we have to do is to remove one of the red axes and its
perpendicular outer blue Decagon, together with their vertices and all the struts that
connect to their vertices. You will then see an alignment of 10 Pentagonal antiprisms
going through the center - in this Figure, we're looking through these. Along this
direction, the Pentagonal antiprisms get progressively flattened as they approach the
outer regions. In the perpendicular ring, another 10 Pentagonal antiprisms are seen
``sideways'', and completely flattened.
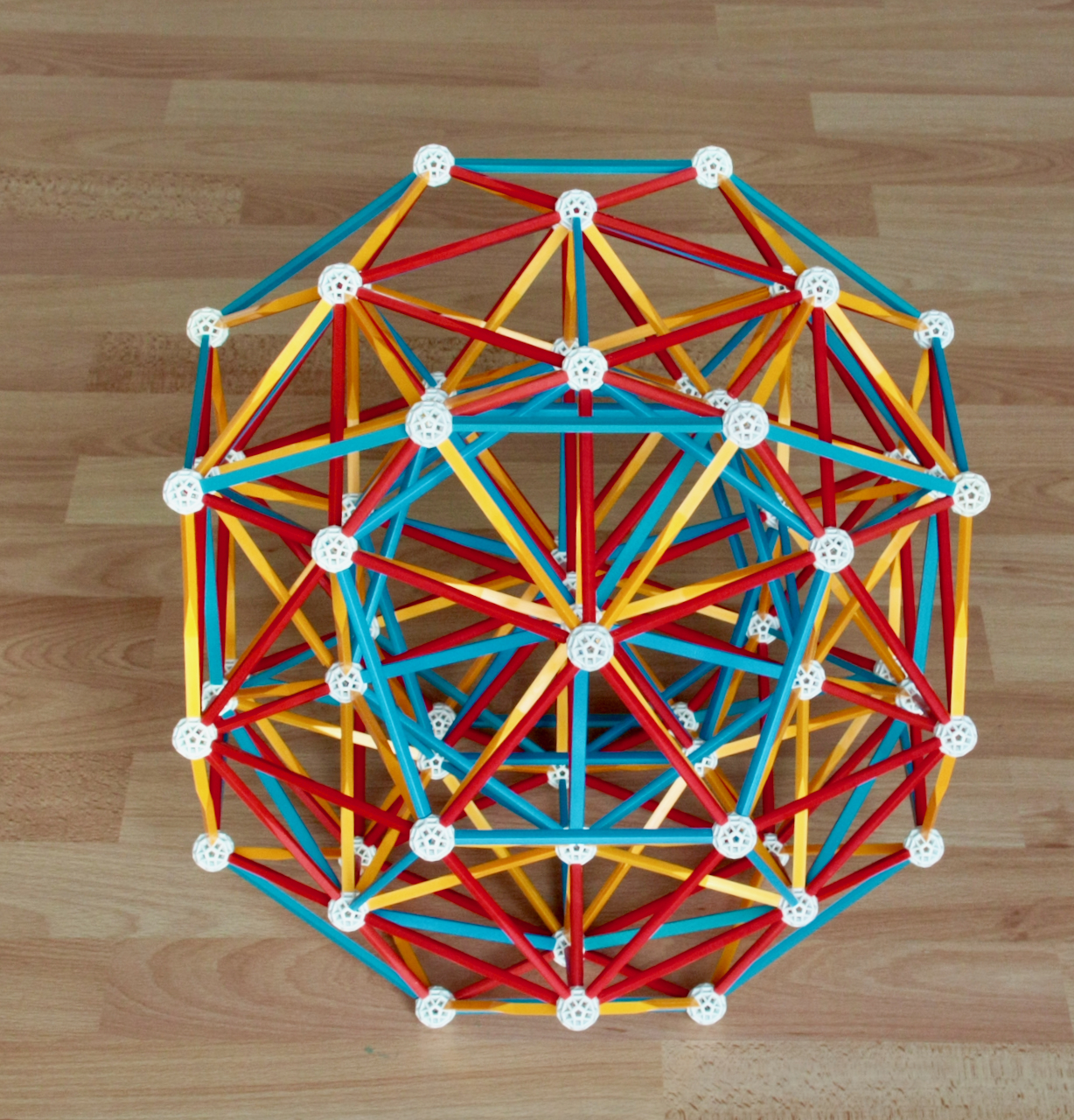
Fig. A.11b: Here is the second Zomable projection of the Grand antiprism!
How to Build: You can see a vZome model of this projection at the end of this page.
The derivation is the same as for Fig. A.11a, but using instead the other two types of
Decagons that appear in the projection of the 600-cell: one type has its edges coinciding
with the edges of the inner blue Icosahedron (connecting to the vertices of the outer blue
Icosidodecahedron via short red struts) and a perpendicular one, with edges coinciding
with the blue Dodecahedron, which connect with the vertices of the outer blue
Icosidodecahedron via yellow struts. Apart from these and those missing in the model in
Fig. A.11a, there are no additional types of Decagons in the Icosahedral projection of the
600-cell.
See also Eusebeia page on the Grand
antiprism.
Its structure also helps understanding the structure of the 600-cell, in particular the
fact that the latter can be decomposed in two disjoint perpendicular rings of 150
Tetrahedra each (which are "fused" to make the rings of 10 Pentagonal Antiprisms of the
Grand antiprism), separated by a Clifford torus of 100 Tetrahedra
with the two sets of 100 Tetrahedra that border that torus on each side.
Zomable Archimedean polychora and Triality
We now investigate which Archimedean polychora are Zomable:
- All 9 Archimedean
polychora derived from the symmetry of the 5-cell are Zomable - and in multiple
ways, one for each projection of the 5-cell in Figs. 5.3 and 7.8. In this site, we
presented three of these polychora:
- The 5-cell (Fig. 5.3, 7.8),
- The Rectified 5-cell (Fig. 5.3),
- The Omnitruncated 5-cell (Fig. A.10).
- Furthermore, all 15 Archimedean polychora derived from the symmetry of the 600-cell
are Zomable, with
all projections having Icosahedral symmetry, and all five being Zomable with B, R and Y
struts. In this site we presented five of these polychora:
- The 600-cell (Fig. 5.10),
- The 120-cell (Fig. 5.11),
- The Rectified 600-cell (Fig. A.4),
- The Rectified 120-cell (Fig. A.5),
- The Truncated 600-cell (Figs. A.9a and b).
- As we've seen, the Grand antiprism is Zomable, a consequence of it being a diminished
600-cell (Figs. A.11a, b).
- However, only 9 of the 22 Archimedean polychora built with the symmetries of the
16-cell and 24-cell are Zomable: all others have Octagons, either in cells or edge
sections. All nine Zomable polychora appear in this site, all nine are necessarily built
with the Zomable cells in Fig. A.2:
- The 16-cell (Fig. 5.4),
- The Tesseract (Fig. 5.4),
- The 24-cell (Figs. 5.5a and b),
- The Rectified 24-cell (Fig. 5.6),
- The Snub 24-cell (Fig. 5.8),
- The Rectified tesseract (Figs. A.3a and b),
- The Truncated 16-cell (Figs. A.6a and b),
- The Bitruncated 16-cell (Figs. A.7a and b),
- The Truncated 24-cell (Fig. A.8).
Interestingly, these Zomable polychora include all four cases that are generated from both
the symmetries of the 16-cell and 24-cell (the "double counts" in the enumeration above),
these have "24-cell" in their names. No additional polychora generated with the symmetry
of the 24-cell are Zomable.
Even more interesting is the fact that, apart from the Tesseract, the latter set of
Zomable polychora is the full set of polychora generated by
the demi-tesseractic symmetry, which is the 4-dimensional demi-hypercubic symmetry.
Therefore, of all Archimedean polychora generated from the symmetry of the 16-cell and
24-cell, only the full set of ``Demi-tesseractic'' polychora are Zomable, plus the
Tesseract! This situation is similar to that of the Archimedean solids with Tetrahedral
and Octahedral symmetry, where only the full set of polyhedra derived from the demi-cubic
symmetry (the Tetrahedral symmetry, which is the 3-dimensional demi-hypercubic symmetry, in
green in Fig. A.2), plus the Cube (in blue in Fig. A.2) are Zomable.
Why the exceptions for the Tesseract and the Cube? As we've seen for the facetings of the 24-cell, the edges
of the Tesseract are a subset of the edges of the 24-cell, so if the latter is Zomable, so
is the former. The same applies therefore to its cells, all Cubic. It is logically
necessary that the Cube is Zomable, because it is a cell not only of the Tesseract but
also of two demi-tesseractic polychora, the Rectified 24-cell and the Truncated 24-cell.
***
Interestingly, the demi-tesseractic symmetry is the one that exhibits the phenomenon of triality. This means that the
Demi-tesseractic polychora are not only Zomable, but they have many Zomable projections!
We now investigate these.
Those derived from the symmetry of the 16-cell have three sets of Zomable ``trial''
projections, like the 16-cell itself, these include the green-blue projections shown above
(which can be derived from the projections of the 16-cell in Fig. 5.4), three orthogonal
pyritohedric projections (like the projections of the 16-cell in Fig. 7.1a) and three
trial prismatic projections (like the projections of the 16-cell in Fig. 7.1b). These
projections were calculated using vZome.
The blue-green projections of the Rectified tesseract are shown in Figs. A.3a and b.
Below we show all remaining projections.

Fig. A.12a: The pyritohedric projection of the Rectified tesseract. This is centred on a
Square face. The two other trial projections would have these central Squares in
orthogonal planes, like the central edges of the 16-cell in Fig. 7.1a.

Fig. A.12b: One trial prismatic projection of the Rectified tesseract.
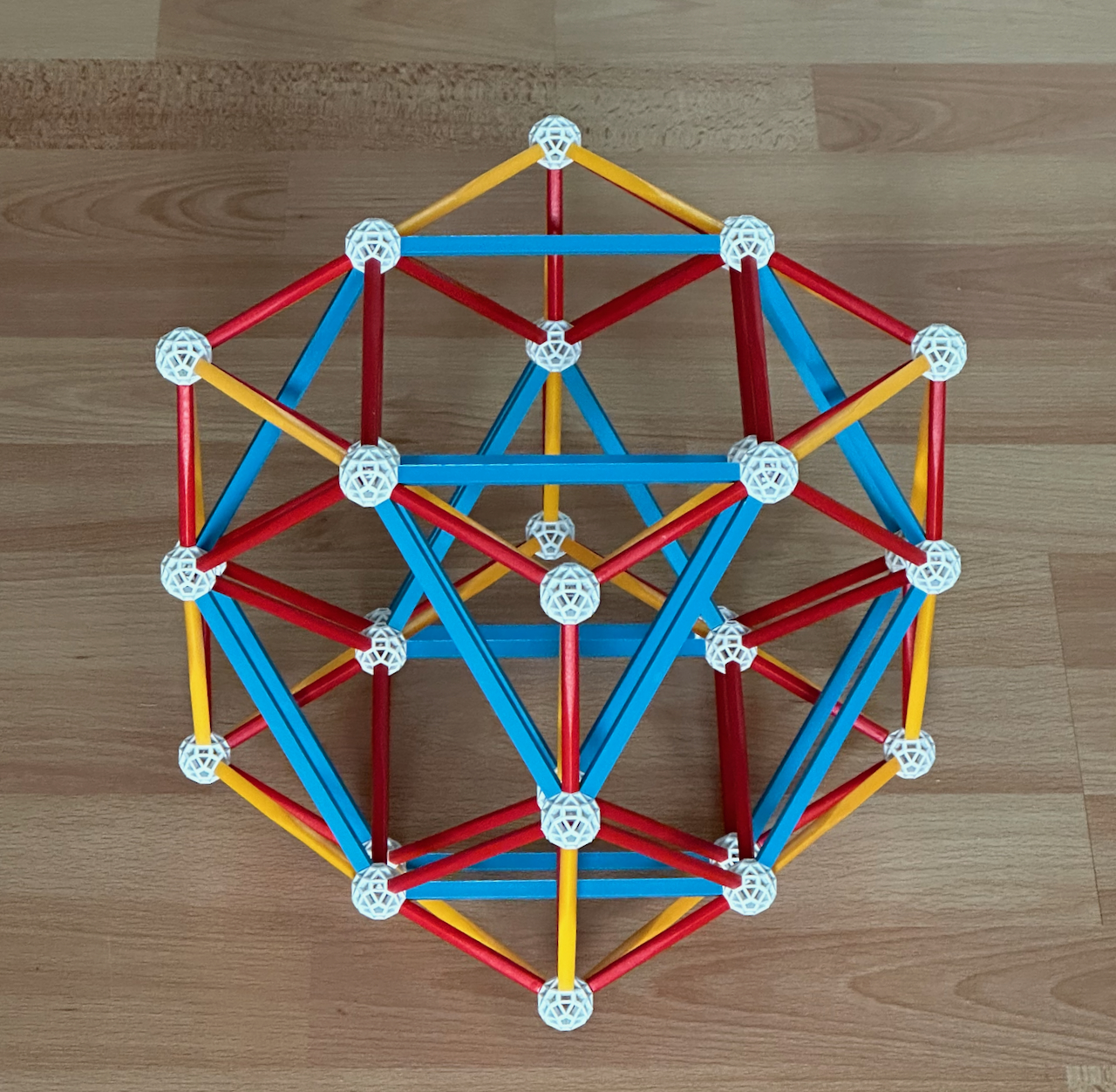
Fig. A.12c: Another trial prismatic projection of the Rectified tesseract.

Fig. A.12d: Another trial prismatic projection of the Rectified tesseract.
All three trial projections require 32 connectors, 24 B2 struts, 24 R2 struts, 24 Y2
struts and 24 R1 struts.
The blue-green projections of the Truncated 16-cell are shown in Figs. A.6a and b.
Below we show all remaining projections.

Fig. A.13a: The pyritohedric projection of the Truncated 16-cell. Like the pyritohedric
projection of the 16-cell, this is centred on an edge. The two other trial projections
would have these central edges in orthogonal axes, like the central edges of the
16-cell in Fig. 7.1a. Note the similarity with Fig. A.6c.

Fig. A.13b: One trial prismatic projection of the Truncated 16-cell.

Fig. A.13c: Another trial prismatic projection of the Truncated 16-cell.
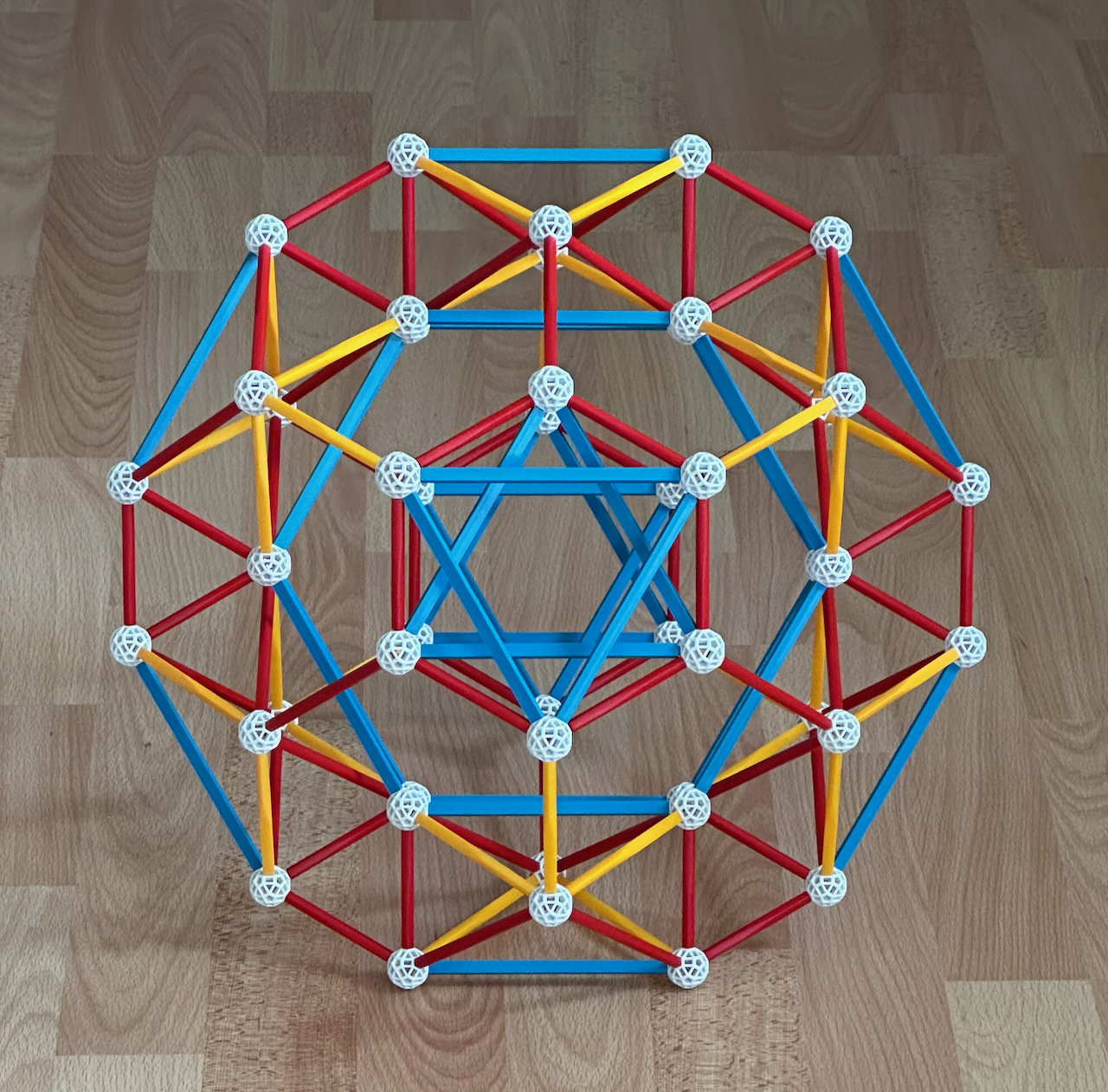
Fig. 13d: Another trial prismatic projection of the Truncated 16-cell.
The three trial projections require 48 connectors, 30 B2 struts, 30 R2 struts, 30 Y2
struts and 30 R1 struts.
These projections are truncations of the projections of the 16-cell that appear in Figs.
7.1a and b.
David
Richter's detailed explanation of Triality shows the prismatic projections of the 16
cell and truncated 16-cell from a different perspective.
The blue-green projections of the Bitruncated 16-cell are shown in Figs. A.7a and b.
Below we show all remaining projections.
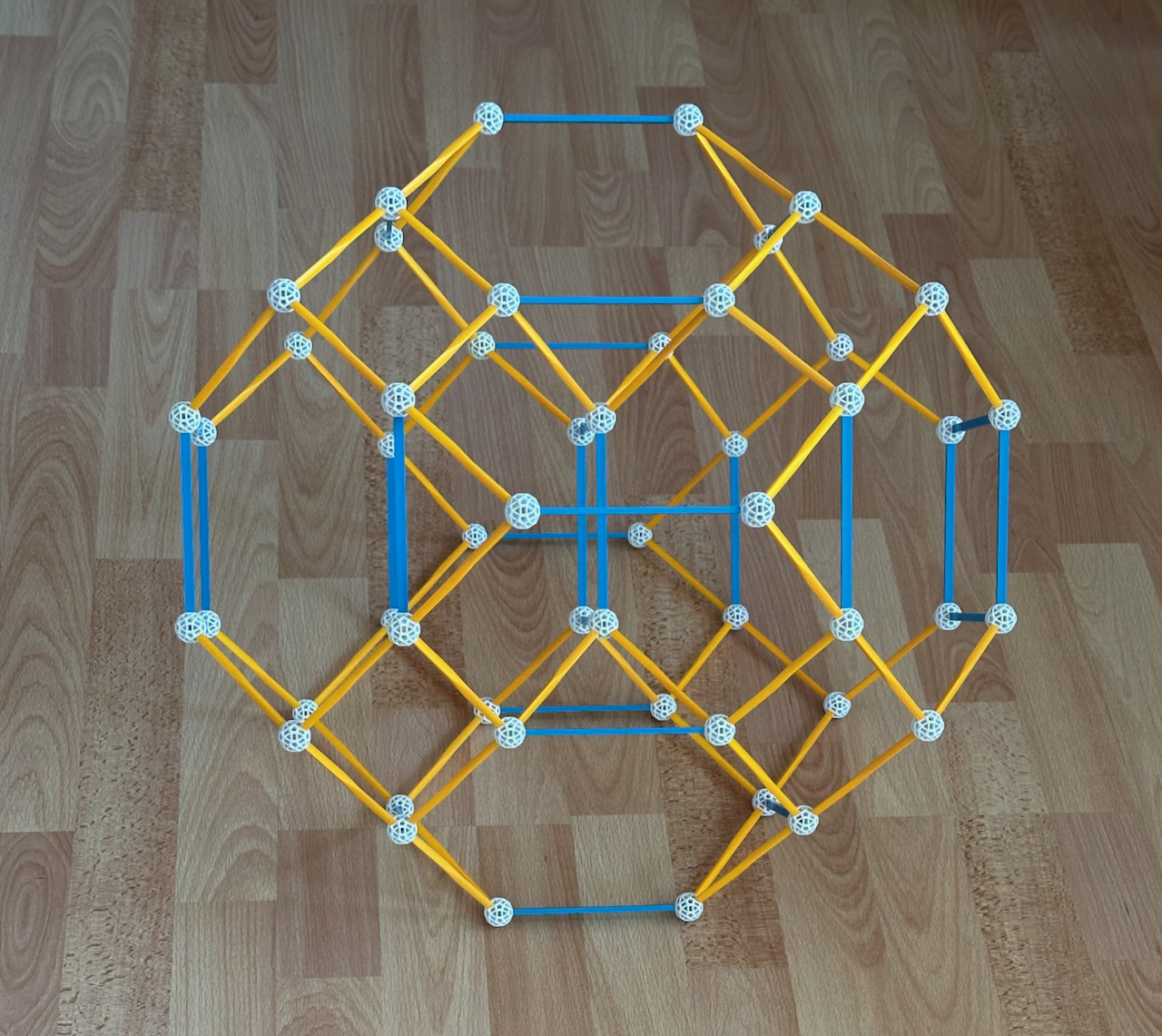
Fig. A.14a: The pyritohedric projection of the Bitruncated 16-cell/Tesseract. Like the
pyritohedric projection of the Rectified tesseract, this is centred on an Square face. The
two other trial projections would have these central Squares in orthogonal planes, like
the central edges of the 16-cell in Fig. 7.1a. Note the similarity with Fig. A.7c.

Fig. A.14b: One trial prismatic projection of the Bitruncated 16-cell/Tesseract.

Fig. A.14c: Another trial prismatic projection of the Bitruncated 16-cell/Tesseract.
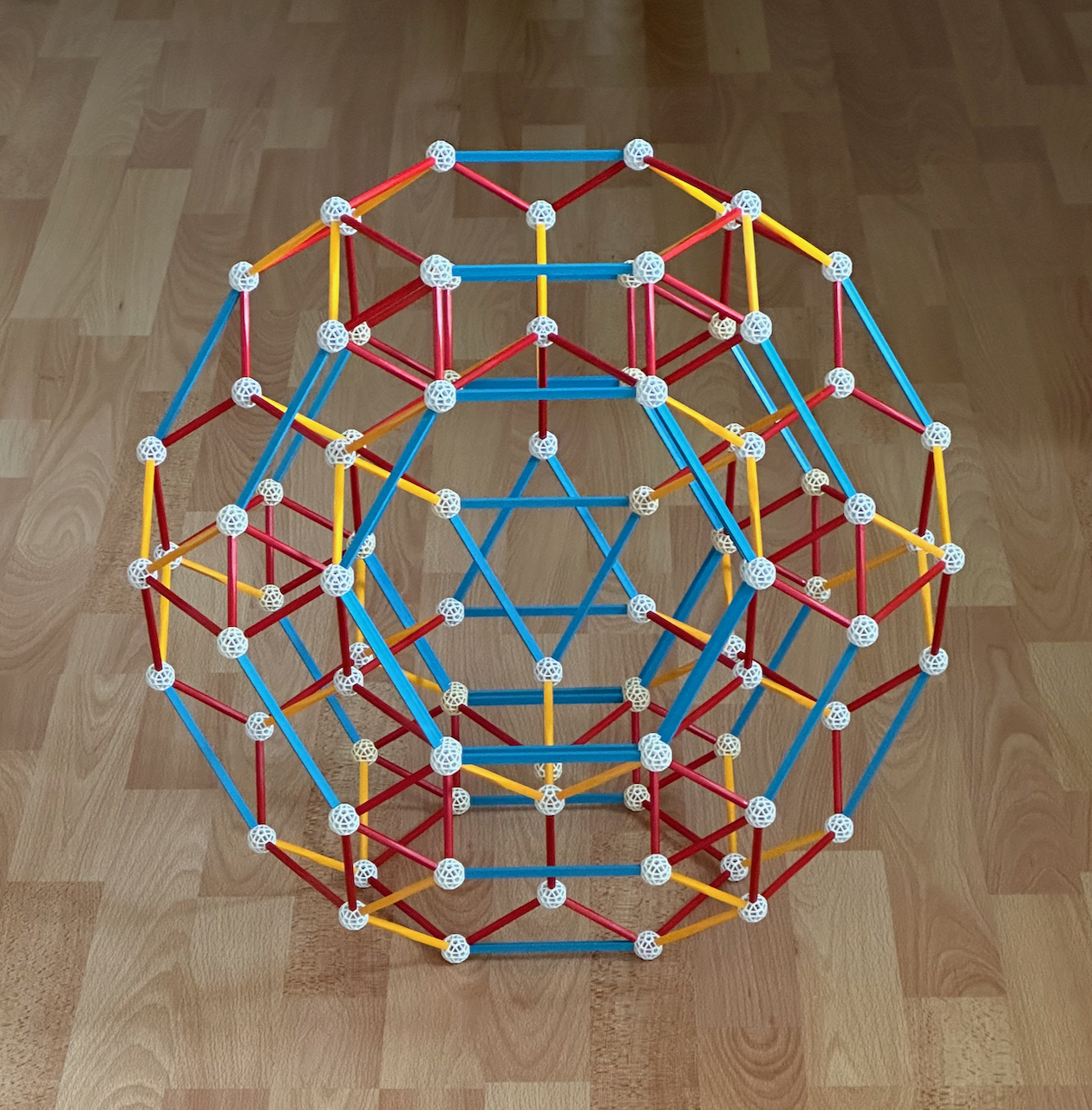
Fig. A.14d: Another trial prismatic projection of the Bitruncated 16-cell/Tesseract.
The three trial projections require 96 connectors, 48 B2 struts, 48 R2 struts, 48 Y2
struts and 48 R1 struts.
The demi-tesseractic polychora derived from the 24-cell are special, because they can be
derived from the symmetries of the 16-cell, 24-cell and the demi-tesseractic symmetry.
Apart from the Snub 24-cell, the others have three Zomable projections each, like the
24-cell: Apart from the green-blue projections (Figs. 5.5a, 5.6 and A.8), these include a
pyritohedric and a prismatic projection, which correspond to the projections of the
24-cell in Figs. 7.1a and b.
The blue-green projection of the Rectified 24-cell is shown in Fig. 5.6. Below we show the
two remaining projections.

Fig. A.15a: The pyritohedric projection of the Rectified 24-cell. This is the rectification
of the pyritohedric projection of the 24-cell in Fig. 5.5b, and like it it has full
Octahedral symmetry. It is centred on a Cube.
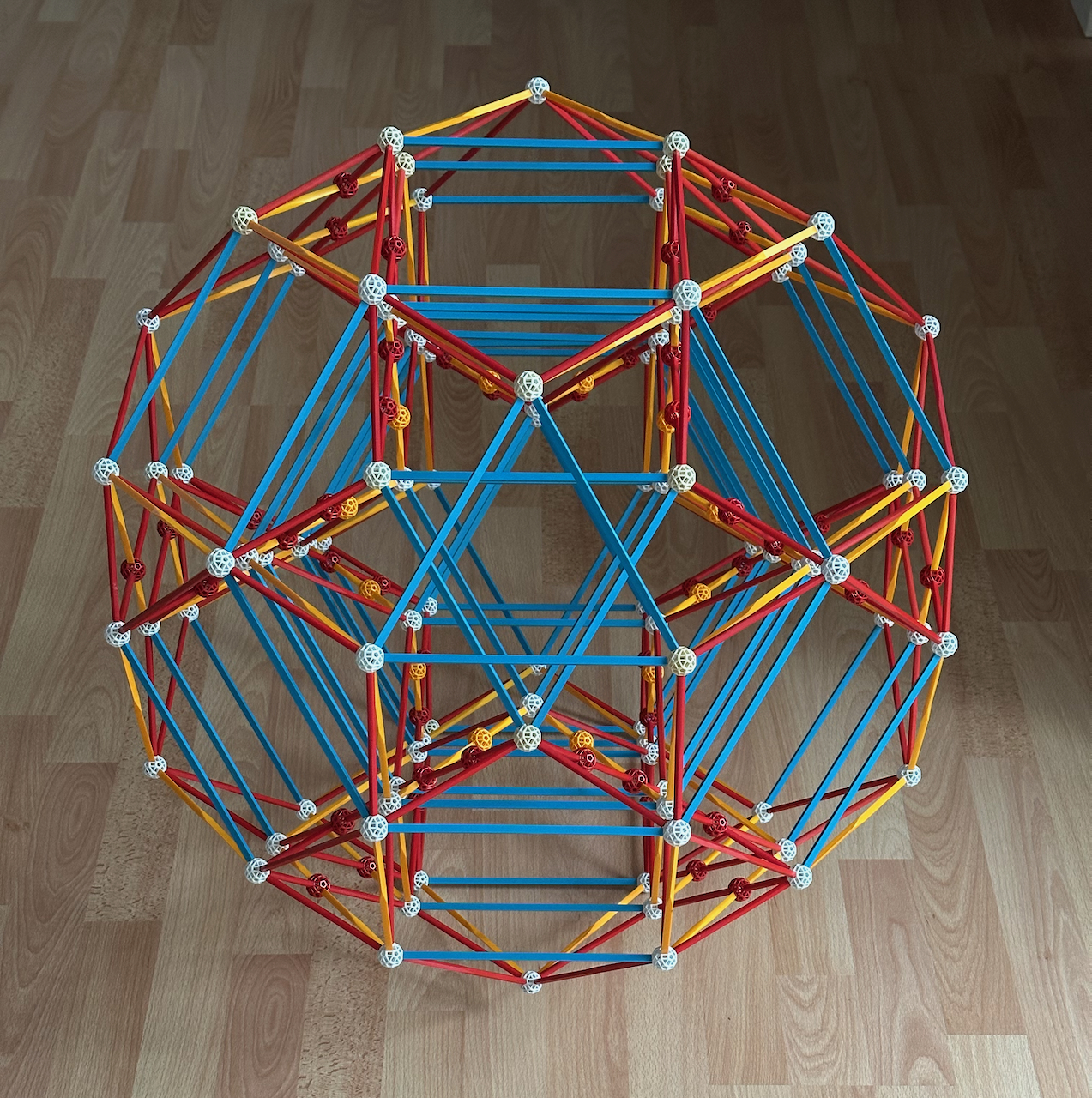
Fig. A.15b: The prismatic projection of the Rectified 24-cell. This is the rectification
of the prismatic projection of the 24-cell in Fig. 7.1b, and like it it has the same
antiprismatic symmetry. Here it is seen from its 3-fold symmetry axis. Notice the
similarity with Fig. 5.6.
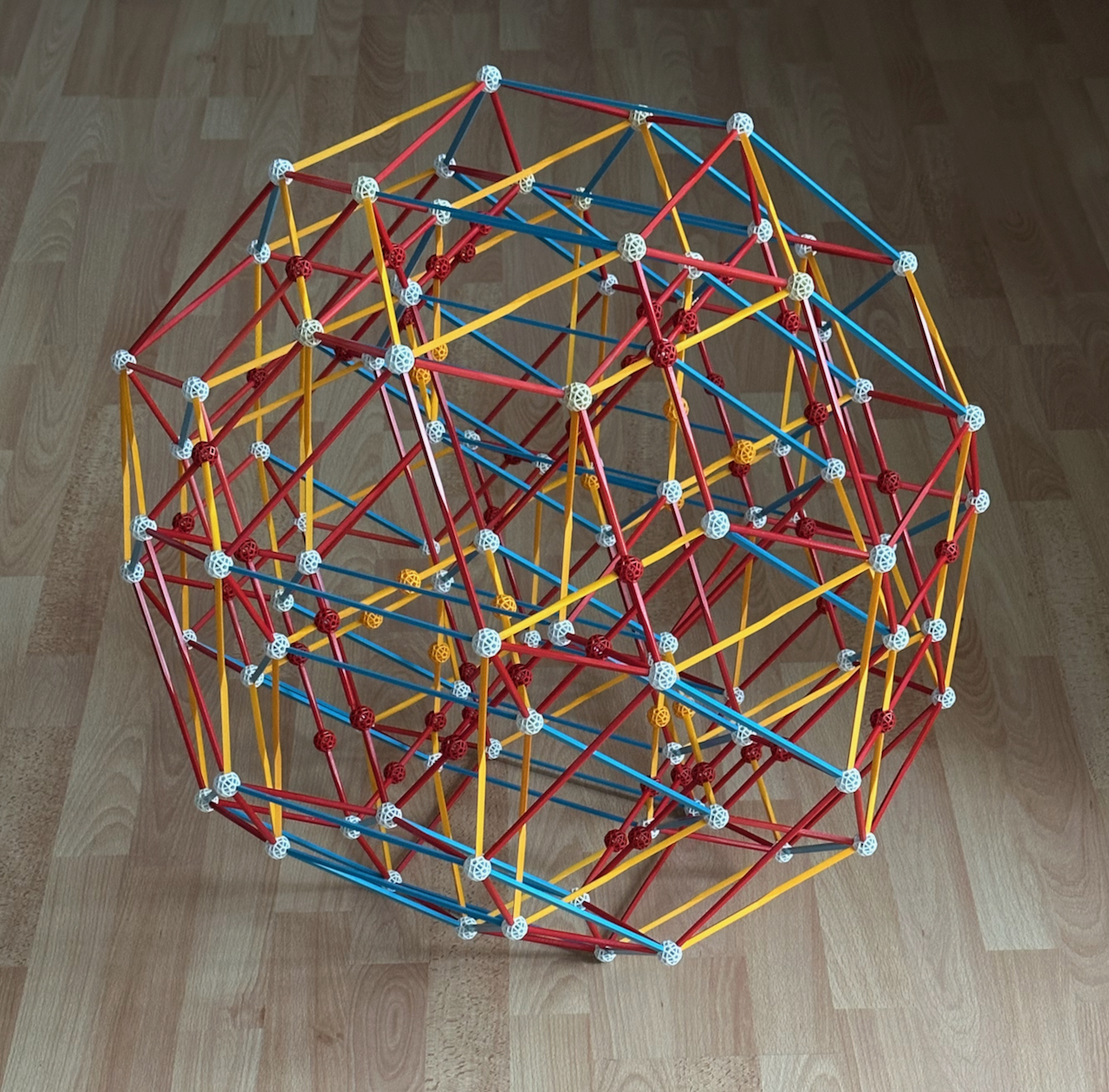
Fig. A.15c: The same model, seen from a generic perspective.
The prismatic projection requires, 96 connectors to represent the 96 vertices of the
polychoron and, theoretically, 72 B3 struts, 72 R3 struts, 72 Y3 struts and 72 R2 struts,
which represent its 288 edges, all of which appear without any superpositions. However, as
in the case of the prismatic projection of the 24-cell, there are several edge
intersections that happen only in the projection, not in the polychoron. Because of these,
several R3 and Y3 struts are broken into sets of smaller struts, which include Y0 struts.
For this reason the model cannot be built on a smaller scale.
The blue-green projection of the Truncated 24-cell is shown in Fig. A.8. Below we show the
pyritohedric projection. In the prismatic projection of this polychoron there are several
intersections between red and yellow parts that are difficult to implement at a reasonable
scale, for this reason I did not build it.

Fig. A.16: The pyritohedric projection of the Truncated 24-cell. This is the truncation of
the pyritohedric projection of the 24-cell in Fig. 5.5b, and like it it has full
Octahedral symmetry. Like the previous pyritohedric projection (Fig. A.15a), it is also
centred on a Cube.
To conclude, we note the Wythoff construction can be applied to the flat analog of the
regular polychora, the Cubic honeycomb. This results in
many of the Archimedean honeycombs,
the flat analogs of the Archimedean polychora, but not all, as several are Non-Wythoffian. These honeycombs are of practical interest for
fields like architecture, engineering and crystallography.
As for polyhedra, relaxing the condition of convexity results in many more ``Archimedean''
star polychora, with more than 2000 known
forms! These include the regular
and partially regular star
polychora as special cases.
Paulo's polytope site.


































